Advertisements
Advertisements
प्रश्न
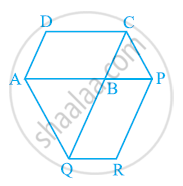
In the given figure, AP || BQ || CR. Prove that ar (AQC) = ar (PBR).

उत्तर
Since ΔABQ and ΔPBQ lie on the same base BQ and are between the same parallels AP and BQ,
∴ Area (ΔABQ) = Area (ΔPBQ) ... (1)
Again, ΔBCQ and ΔBRQ lie on the same base BQ and are between the same parallels BQ and CR.
∴ Area (ΔBCQ) = Area (ΔBRQ) ... (2)
On adding equations (1) and (2), we obtain
Area (ΔABQ) + Area (ΔBCQ) = Area (ΔPBQ) + Area (ΔBRQ)
⇒ Area (ΔAQC) = Area (ΔPBR)
APPEARS IN
संबंधित प्रश्न
XY is a line parallel to side BC of a triangle ABC. If BE || AC and CF || AB meet XY at E and F respectively, show that
ar (ABE) = ar (ACF)
The side AB of a parallelogram ABCD is produced to any point P. A line through A and parallel to CP meets CB produced at Q and then parallelogram PBQR is completed (see the following figure). Show that
ar (ABCD) = ar (PBQR).
[Hint: Join AC and PQ. Now compare area (ACQ) and area (APQ)]
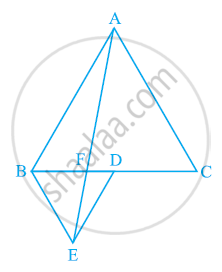
In the given figure, ABCDE is a pentagon. A line through B parallel to AC meets DC produced at F. Show that
(i) ar (ACB) = ar (ACF)
(ii) ar (AEDF) = ar (ABCDE)

In the following figure, ABC and BDE are two equilateral triangles such that D is the mid-point of BC. If AE intersects BC at F, show that
(i) ar (BDE) = 1/4 ar (ABC)
(ii) ar (BDE) = 1/2 ar (BAE)
(iii) ar (ABC) = 2 ar (BEC)
(iv) ar (BFE) = ar (AFD)
(v) ar (BFE) = 2 ar (FED)
(vi) ar (FED) = 1/8 ar (AFC)
[Hint : Join EC and AD. Show that BE || AC and DE || AB, etc.]
If a triangle and a parallelogram are on the same base and between same parallels, then the ratio of the area of the triangle to the area of parallelogram is ______.
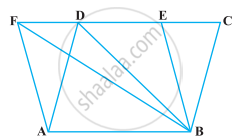
The area of the parallelogram ABCD is 90 cm2 (see figure). Find
- ar (ΔABEF)
- ar (ΔABD)
- ar (ΔBEF)
The area of the parallelogram ABCD is 90 cm2 (see figure). Find ar (ΔBEF)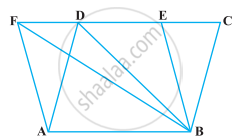
A point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar (ADF) = ar (ABFC)
The medians BE and CF of a triangle ABC intersect at G. Prove that the area of ∆GBC = area of the quadrilateral AFGE.
In ∆ABC, if L and M are the points on AB and AC, respectively such that LM || BC. Prove that ar (LOB) = ar (MOC)
