Advertisements
Advertisements
प्रश्न
In the figure, if ∠ACB = ∠CDA, AC = 8 cm and AD = 3 cm, find BD.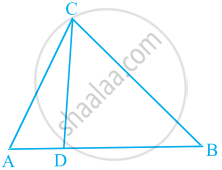
उत्तर
Given,
AC = 8 cm,
AD = 3 cm
And ∠ACB = ∠CDA
From figure,
∠CDA = 90°
∠ACB = ∠CDA = 90°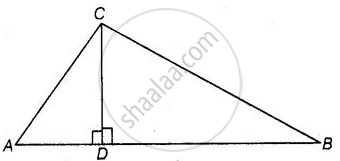
In right angled ΔADC,
AC2 = AD2 + CD2
⇒ (8)2 = (3)2 + (CD)2
⇒ 64 – 9 = CD2
⇒ CD = `sqrt(55)` cm
In ΔCDB and ΔADC,
∠BDC = ∠ADC ...[Each 90°]
∠DBC = ∠DCA ...[Each equal to 90° – ∠A]
∴ ΔCDB ∼ ΔADC
Then, `("CD")/("BD") = ("AD")/("CD")`
⇒ CD2 = AD × BD
∴ BD = `("CD"^2)/("AD")`
= `(sqrt(155))^2/3`
= `55/3` cm
APPEARS IN
संबंधित प्रश्न
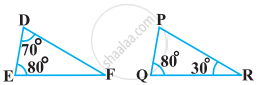
State which pair of triangles in the following figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE ∼ ΔCFB.
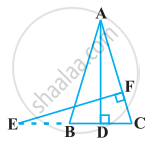
In the following figure, E is a point on side CB produced of an isosceles triangle ABC with AB = AC. If AD ⊥ BC and EF ⊥ AC, prove that ΔABD ∼ ΔECF.
A vertical pole of a length 6 m casts a shadow 4m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
In the following figure, AB || QR. Find the length of PB.

In ΔABC~ΔDEF such that 2AB = DE and BC = 6cm, find EF.
In ABC, DE || AB. If CD = 3 cm, EC = 4 cm, BE = 6 cm, then DA is equal to ______.
It is given that ∆ABC ~ ∆EDF such that AB = 5 cm, AC = 7 cm, DF = 15 cm and DE = 12 cm. Find the lengths of the remaining sides of the triangles.
In the figure with ΔABC, P, Q, R are the mid-points of AB, AC and BC respectively. Then prove that the four triangles formed are congruent to each other.

Which of the following is NOT a similarity criterion of traingles?
