Advertisements
Advertisements
प्रश्न
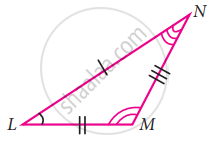
In the following example, a pair of triangles is shown. Equal parts of triangle in each pair are marked with the same sign. Observe the figure and state the test by which the triangle in each pair are congruent.

By ______ test
ΔXYZ ≅ ΔLMN
उत्तर
By SAS Test
APPEARS IN
संबंधित प्रश्न
BD and CE are bisectors of ∠B and ∠C of an isosceles ΔABC with AB = AC. Prove that BD = CE.
Find the measure of each angle of an equilateral triangle.
In the given figure, the measure of ∠B'A'C' is

If ABC and DEF are two triangles such that ΔABC \[\cong\] ΔFDE and AB = 5cm, ∠B = 40°
Prove that:
(i) ∆ ABC ≅ ∆ ADC
(ii) ∠B = ∠D
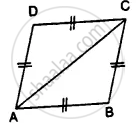
In the figure, ∠CPD = ∠BPD and AD is the bisector of ∠BAC. Prove that ΔCAP ≅ ΔBAP and CP = BP.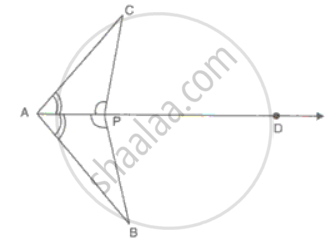
ΔABC is isosceles with AB = AC. BD and CE are two medians of the triangle. Prove that BD = CE.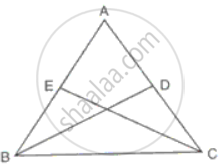
If the given two triangles are congruent, then identify all the corresponding sides and also write the congruent angles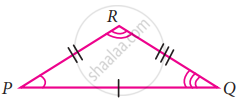
“If two sides and an angle of one triangle are equal to two sides and an angle of another triangle, then the two triangles must be congruent.” Is the statement true? Why?
The top and bottom faces of a kaleidoscope are congruent.
