Advertisements
Advertisements
प्रश्न
In the given figure, P is a point on AB such that AP : PB = 4 : 3. PQ is parallel to AC.
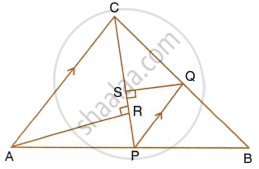
- Calculate the ratio PQ : AC, giving reason for your answer.
- In triangle ARC, ∠ARC = 90° and in triangle PQS, ∠PSQ = 90°. Given QS = 6 cm, calculate the length of AR.
उत्तर
i. Given, AP : PB = 4 : 3.
Since, PQ || AC.
Using Basic Proportionality theorem,
`(AP)/(PB) = (CQ)/(QB)`
`=> (CQ)/(QB) = 4/3`
`=> (BQ)/(BC) = 3/7` ...(1)
Now, ∠PQB = ∠ACB ...(Corresponding angles)
∠QPB = ∠CAB ...(Corresponding angles)
∴ ΔPBQ ~ ΔABC ...(AA similarity)
`=> (PQ)/(AC) = (BQ)/(BC)`
`=> (PQ)/(AC) = 3/7` ...[Using (1)]
ii. ∠ARC = ∠QSP = 90°
∠ACR = ∠SPQ ...(Alternate angles)
∴ ∆ARC ~ ∆QSP ...(AA similarity)
`=> (AR)/(QS) = (AC)/(PQ)`
`=> (AR)/(QS) = 7/3`
`=> AR = (7 xx 6)/3 = 14 cm`
APPEARS IN
संबंधित प्रश्न
In ∆ ABC, ∠B = 2 ∠C and the bisector of angle B meets CA at point D. Prove that:
(i) ∆ ABC and ∆ ABD are similar,
(ii) DC: AD = BC: AB
In ∆ABC, right – angled at C, CD ⊥ AB.
Prove:
`"CD"^2 = "AD"xx "DB"`
Given : AB || DE and BC || EF. Prove that :
- `(AD)/(DG) = (CF)/(FG)`
- ∆DFG ∼ ∆ACG
In the given figure, AX : XB = 3 : 5
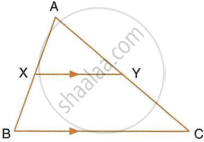
Find:
- the length of BC, if the length of XY is 18 cm.
- the ratio between the areas of trapezium XBCY and triangle ABC.
In the given figure, ∠B = ∠E, ∠ACD = ∠BCE, AB = 10.4 cm and DE = 7.8 cm. Find the ratio between areas of the ∆ABC and ∆DEC.
In the following diagram, lines l, m and n are parallel to each other. Two transversals p and q intersect the parallel lines at points A, B, C and P, Q, R as shown.
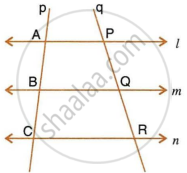
Prove that : `(AB)/(BC) = (PQ)/(QR)`
In the given figure, triangle ABC is similar to triangle PQR. AM and PN are altitudes whereas AX and PY are medians.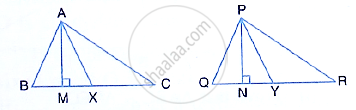 Prove that : `(AM)/(PN)=(AX)/(PY)`
Prove that : `(AM)/(PN)=(AX)/(PY)`
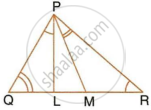
In a triangle PQR, L and M are two points on the base QR, such that ∠LPQ = ∠QRP and ∠RPM = ∠RQP. Prove that:
- ΔPQL ∼ ΔRPM
- QL × RM = PL × PM
- PQ2 = QR × QL
The following figure shows a triangle ABC in which AD and BE are perpendiculars to BC and AC respectively.
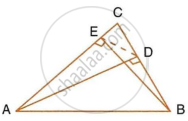
Show that:
- ΔADC ∼ ΔBEC
- CA × CE = CB × CD
- ΔABC ~ ΔDEC
- CD × AB = CA × DE
In the given figure, ABC is a right angled triangle with ∠BAC = 90°.
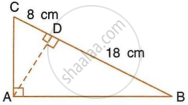
- Prove that : ΔADB ∼ ΔCDA.
- If BD = 18 cm and CD = 8 cm, find AD.
- Find the ratio of the area of ΔADB is to area of ΔCDA.
