Advertisements
Advertisements
प्रश्न
In young’s double slit experiment, deduce the conditions for obtaining constructive and destructive interference fringes. Hence, deduce the expression for the fringe width.
उत्तर
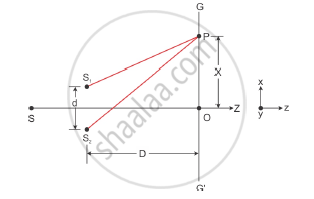
Young’s double slit experiment demonstrated the phenomenon of interference of light. Consider two fine slits S1 and S2 at a small distance d apart. Let the slits be illuminated by a monochromatic source of light of wavelength λ. Let GG′ be a screen kept at a distance D from the slits. The two waves emanating from slits S1 and S2 superimpose on each other resulting in the formation of an interference pattern on the screen placed parallel to the slits.
Let O be the centre of the distance between the slits. The intensity of light at a point on the screen will depend on the path difference between the two waves reaching that point. Consider an arbitrary point P at a distance x from O on the screen.
Path difference between two waves at P = S2P − S1P
The intensity at the point P is maximum or minimum as the path difference is an integral multiple of wavelength or an odd integral multiple of half wavelength
For the point P to correspond to maxima, we must have
S2P − S1P = n, n = 0, 1, 2, 3...
From the figure given above
`(S_2P)^2-(S_1P)^2=D^2+(x+d/2)^2-D^2+(x-d/2)^2`
On solving we get:
(S2P)2-(S1P)2=2xd
`S_2P-S_1P=(2xd)/(S_2P+S_1P)`
As d<<D, then S2P + S2P = 2D (∵ S1P = S2P ≡ D when d<<D)
`:.S_2P-S_1P=(2xd)/(2D)=(xd)/D`
Path difference, `S_2P-S_1P=(xd)/D`
Hence, when constructive interfernce occur, bright region is formed.
For maxima or bright fringe, path difference = `xd/D=nlambda`
i.e `x=(nlambdaD)/d`
where n=0,± 1, ±2,........
During destructive interference, dark fringes are formed:
Path difference, `(xd)/D=(n+1/2)lambda`
`x=(n+1/2)(lambdaD)/d`
The dark fringe and the bright fringe are equally spaced and the distance between consecutive bright and dark fringe is given by:
β = xn+1-xn
`beta=((n+1)lambdaD)/d-(nlambdaD)/d`
`beta=(lambdaD)/d`
Hence the fringe width is given by `beta = (lambdaD)/d`
APPEARS IN
संबंधित प्रश्न
The intensity at the central maxima in Young’s double slit experiment is I0. Find out the intensity at a point where the path difference is` lambda/6,lambda/4 and lambda/3.`
Find the intensity at a point on a screen in Young's double slit experiment where the interfering waves have a path difference of (i) λ/6, and (ii) λ/2.
In Young's double slit experiment, derive the condition for
(i) constructive interference and
(ii) destructive interference at a point on the screen.
How does an unpolarized light incident on a polaroid get polarized? Describe briefly, with the help of a necessary diagram, the polarization of light by reflection from a transparent medium.
Can we perform Young's double slit experiment with sound waves? To get a reasonable "fringe pattern", what should be the order of separation between the slits? How can the bright fringes and the dark fringes be detected in this case?
Consider the arrangement shown in the figure. By some mechanism, the separation between the slits S3 and S4 can be changed. The intensity is measured at the point P, which is at the common perpendicular bisector of S1S2 and S2S4. When \[z = \frac{D\lambda}{2d},\] the intensity measured at P is I. Find the intensity when z is equal to
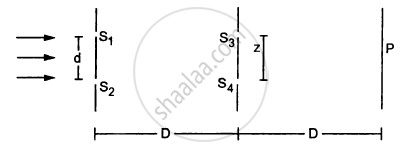
(a) \[\frac{D\lambda}{d}\]
(b) \[\frac{3D\lambda}{2d}\] and
(c) \[\frac{2D\lambda}{d}\]
In Young's double slit experiment using monochromatic light of wavelength 600 nm, 5th bright fringe is at a distance of 0·48 mm from the centre of the pattern. If the screen is at a distance of 80 cm from the plane of the two slits, calculate:
(i) Distance between the two slits.
(ii) Fringe width, i.e. fringe separation.
The force required to double the length of a steel wire of area 1 cm2, if its Young's modulus Y= 2 × 1011/m2 is:
In Young's double slit experiment, the minimum amplitude is obtained when the phase difference of super-imposing waves is: (where n = 1, 2, 3, ...)
The maximum number of possible interference maxima for slit-separation equal to twice the wavelength in Young's double-slit experiment is ______.
