Advertisements
Advertisements
प्रश्न
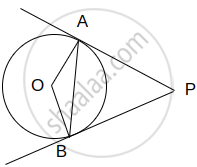
PA and PB are tangents drawn to a circle of centre O from an external point P. Chord AB makes an angle of 30° with the radius at the point of contact. If length of the chord is 6 cm, find the length of the tangent PA and the length of the radius OA.
उत्तर
∠OAB = 30°
∠OAP = 90° ...[Angle between the tangent and the radius at the point of contact]
∠PAB = 90° – 30° = 60°
AP = BP ...[Tangents to a circle from an external point]
∠PAB = ∠PBA ...[Angles opposite to equal sides of a triangle]
In ΔABP, ∠PAB + ∠PBA + ∠APB = 180° ...[Angle Sum Property]
60° + 60° + ∠APB = 180°
∠APB = 60°
∴ ΔABP is an equilateral triangle, where AP = BP = AB.
PA = 6 cm
In Right ΔOAP, ∠OPA = 30°
tan 30° = `(OA)/(PA)`
`1/sqrt(3) = (OA)/6`
OA = `6/sqrt(3) = 2sqrt(3)cm`
APPEARS IN
संबंधित प्रश्न
Prove that a parallelogram circumscribing a circle is a rhombus.
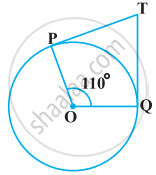
In the given figure, if TP and TQ are the two tangents to a circle with centre O so that ∠POQ = 110°, then ∠PTQ is equal to ______.
In the given circle with center o, ∠ABC=100°, ∠ACD=40° and CT is tangent to the circle at C. find ∠ADC and ∠DCT.

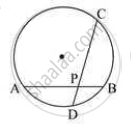
In the given figure PA = 6, PB = 4 and PC = 8. Find PD
Prove that the lengths of two tangent segments drawn to the circle from an external point are equal.
Two concentric circles of radii a and b (a > b) are given. Find the length of the chord of the larger circle which touches the smaller circle.
In fig, O is the centre of the circle, CA is tangent at A and CB is tangent at B drawn to the circle. If ∠ACB = 75°, then ∠AOB = ______

Draw two concentric circles of radii 2 cm and 3 cm. From a point on the outer circle, construct a pair of tangents to the inner circle.
In the given figure, PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q meet at a point T. Find the length of TP.

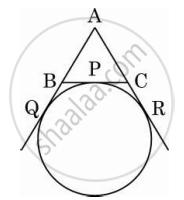
If a circle is touching the side BC of ΔABC at P and is touching AB and AC produced at Q and R respectively (see the figure). Prove that AQ = `1/2` (perimeter of ΔABC).