Advertisements
Advertisements
Question
PA and PB are tangents drawn to a circle of centre O from an external point P. Chord AB makes an angle of 30° with the radius at the point of contact. If length of the chord is 6 cm, find the length of the tangent PA and the length of the radius OA.
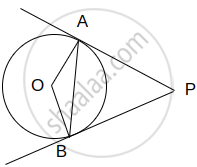
Solution
∠OAB = 30°
∠OAP = 90° ...[Angle between the tangent and the radius at the point of contact]
∠PAB = 90° – 30° = 60°
AP = BP ...[Tangents to a circle from an external point]
∠PAB = ∠PBA ...[Angles opposite to equal sides of a triangle]
In ΔABP, ∠PAB + ∠PBA + ∠APB = 180° ...[Angle Sum Property]
60° + 60° + ∠APB = 180°
∠APB = 60°
∴ ΔABP is an equilateral triangle, where AP = BP = AB.
PA = 6 cm
In Right ΔOAP, ∠OPA = 30°
tan 30° = `(OA)/(PA)`
`1/sqrt(3) = (OA)/6`
OA = `6/sqrt(3) = 2sqrt(3)cm`
APPEARS IN
RELATED QUESTIONS
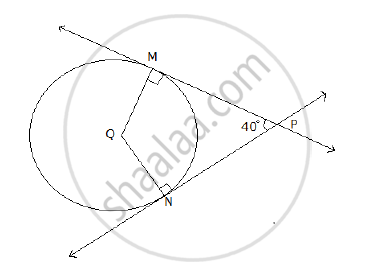
In the following figure, Q is the center of the circle. PM and PN are tangents to the circle. If ∠MPN = 40° , find ∠MQN.
Prove that a parallelogram circumscribing a circle is a rhombus.
A triangle ABC is drawn to circumscribe a circle of radius 4 cm such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 8 cm and 6 cm respectively (see given figure). Find the sides AB and AC.

In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°.
Find:
- ∠OBD
- ∠AOB
- ∠BED

PQ is a tangent drawn from an external point P to a circle with centre O, QOR is the diameter of the circle. If ∠POR = 120°, what is the measure of ∠OPQ?
Construct a pair of tangents to a circle of radius 4 cm from a point which is at a distance of 6 cm from its centre.
The length of the tangent from an external point P on a circle with centre O is ______
If angle between two tangents drawn from a point P to a circle of radius a and centre O is 90°, then OP = `asqrt(2)`.
The length of tangent from an external point P on a circle with centre O is always less than OP.
Draw two concentric circles of radii 2 cm and 5 cm. From a point P on outer circle, construct a pair of tangents to the inner circle.
