Advertisements
Advertisements
प्रश्न
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
उत्तर
We have to prove that the perimeter of a triangle is greater than the sum of its altitude.
In ΔABC
AD⊥ BC , BE ⊥ AC , CF⊥AB

We have to prove
AB + BC + CD > AD + BE + CF
Since AD⊥ BC
So AB > AD and AC > AD
By adding AB + AC > AD + AD, we have
AB + AC > 2AD ........(1)
Now consider BE ⊥ AC then
BC > BE, and BA > BE
Now by adding BC + BA > 2BE .......(2)
Again consider CF⊥AB
AC > CF, and BC > CF
By adding AC + BC > 2CF ...........(3)
Adding (1), (2) and (3), we get
2(AB + BC + CA)>2 (AD + BE + CF)
⇒ AB + BC + CA > AD + BE + CF
Hence the perimeter of a triangle is greater than the sum of all its altitude.
APPEARS IN
संबंधित प्रश्न
Which congruence criterion do you use in the following?
Given: ZX = RP
RQ = ZY
∠PRQ = ∠XZY
So, ΔPQR ≅ ΔXYZ

You have to show that ΔAMP ≅ AMQ.
In the following proof, supply the missing reasons.
| Steps | Reasons | ||
| 1 | PM = QM | 1 | ... |
| 2 | ∠PMA = ∠QMA | 2 | ... |
| 3 | AM = AM | 3 | ... |
| 4 | ΔAMP ≅ ΔAMQ | 4 | ... |

If ΔABC and ΔPQR are to be congruent, name one additional pair of corresponding parts. What criterion did you use?
In two congruent triangles ABC and DEF, if AB = DE and BC = EF. Name the pairs of equal angles.
D, E, F are the mid-point of the sides BC, CA and AB respectively of ΔABC. Then ΔDEF is congruent to triangle
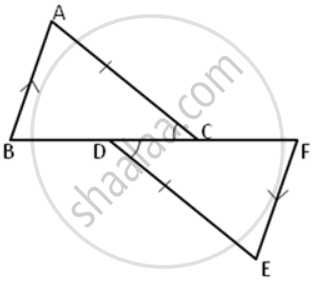
Use the information in the given figure to prove:
- AB = FE
- BD = CF
The following figure shows a circle with center O.
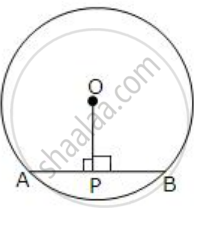
If OP is perpendicular to AB, prove that AP = BP.
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD. Prove that:
AB = CE.
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD. Prove that:
AB is parallel to EC.
In a ΔABC, BD is the median to the side AC, BD is produced to E such that BD = DE.
Prove that: AE is parallel to BC.
