Advertisements
Advertisements
प्रश्न
The following figure shows a circle with center O.
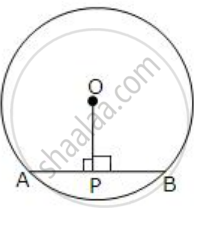
If OP is perpendicular to AB, prove that AP = BP.
उत्तर
Given: In the figure, O is the center of the circle, and AB is a chord. P is a point on AB such that AP=PB.
We need to prove that, AAP=BP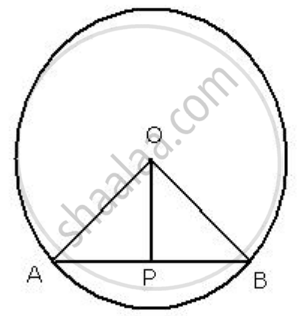
Construction: Join OA and OB
Proof:
In right triangles ΔOAP and ΔOBP
Hypotenuse OA=OB .....[ radii of the same circle ]
Side OP= OP ...[ common ]
∴ By Right Angle- Hypotenuse- Side criterion of congruency, ΔOAP ≅ ΔOBP
The corresponding parts of the congruent triangles are congruent.
∴ AP=BP .....[ by c.p.c.t ]
Hence proved.
APPEARS IN
संबंधित प्रश्न
AD and BC are equal perpendiculars to a line segment AB (See the given figure). Show that CD bisects AB.

In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the given figure). Show that:
- ΔAMC ≅ ΔBMD
- ∠DBC is a right angle.
- ΔDBC ≅ ΔACB
- CM = `1/2` AB

Which congruence criterion do you use in the following?
Given: ZX = RP
RQ = ZY
∠PRQ = ∠XZY
So, ΔPQR ≅ ΔXYZ

Prove that the perimeter of a triangle is greater than the sum of its altitudes.
The given figure shows a circle with center O. P is mid-point of chord AB.

Show that OP is perpendicular to AB.
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD. Prove that:
AB = CE.
In the figure, given below, triangle ABC is right-angled at B. ABPQ and ACRS are squares. 
Prove that:
(i) ΔACQ and ΔASB are congruent.
(ii) CQ = BS.
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
In the following figure, AB = EF, BC = DE and ∠B = ∠E = 90°.
Prove that AD = FC.
In the following figure, ∠A = ∠C and AB = BC.
Prove that ΔABD ≅ ΔCBE. 
