Advertisements
Advertisements
प्रश्न
In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the given figure). Show that:
- ΔAMC ≅ ΔBMD
- ∠DBC is a right angle.
- ΔDBC ≅ ΔACB
- CM = `1/2` AB

उत्तर
Since M is the mid-point of AB.
∴ BM = AM
i. In ΔAMC and ΔBMD, we have
CM = DM ...[Given]
∠AMC = ∠BMD ...[Vertically opposite angles]
AM = BM ...[Proved above]
∴ ΔAMC ≅ ΔBMD ...[By SAS congruency]
ii. Since ΔAMC ≅ ΔBMD
∠MAC = ∠MBD ...[By corresponding parts of congruent triangles]
But they form a pair of alternate interior angles.
∴ AC ‖ DB
Now, BC is a transversal which intersects parallel lines AC and DB,
∴ ∠BCA + ∠DBC = 180° ...[Co-interior angles]
But ∠BCA = 90° ...[ΔABC is right angled at C]
∴ 90° + ∠DBC = 180°
⇒ ∠DBC = 90°
iii. Again, ΔAMC ≅ ΔBMD ...[Proved above]
∴ AC = BD ...[By corresponding parts of congruent triangles]
Now, in ΔDBC and ΔACB, we have
BD = CA ...[Proved above]
∠DBC = ∠ACB ...[Each 90°]
BC = CB ...[Common]
∴ ΔDBC ≅ ΔACB ...[By SAS congruency]
iv. As ΔDBC ≅ ΔACB
⇒ DC = AB ...[By corresponding parts of congruent triangles]
But DM = CM ...[Given]
∴ CM = `1/2` DC = `1/2` AB
⇒ CM = `1/2` AB.
APPEARS IN
संबंधित प्रश्न
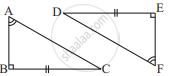
Explain, why ΔABC ≅ ΔFED.
In Δ ABC, ∠B = 35°, ∠C = 65° and the bisector of ∠BAC meets BC in P. Arrange AP, BP and CP in descending order.
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
A triangle ABC has ∠B = ∠C.
Prove that: The perpendiculars from B and C to the opposite sides are equal.
The perpendicular bisectors of the sides of a triangle ABC meet at I.
Prove that: IA = IB = IC.
In the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that: BD = CD

In the following diagram, ABCD is a square and APB is an equilateral triangle.
(i) Prove that: ΔAPD ≅ ΔBPC
(ii) Find the angles of ΔDPC.
In the following diagram, AP and BQ are equal and parallel to each other. 
Prove that: AB and PQ bisect each other.
PQRS is a parallelogram. L and M are points on PQ and SR respectively such that PL = MR.
Show that LM and QS bisect each other.
ABC is a right triangle with AB = AC. Bisector of ∠A meets BC at D. Prove that BC = 2AD.
