Advertisements
Advertisements
प्रश्न
In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B (see the given figure). Show that:
- ΔAMC ≅ ΔBMD
- ∠DBC is a right angle.
- ΔDBC ≅ ΔACB
- CM = `1/2` AB

उत्तर
Since M is the mid-point of AB.
∴ BM = AM
i. In ΔAMC and ΔBMD, we have
CM = DM ...[Given]
∠AMC = ∠BMD ...[Vertically opposite angles]
AM = BM ...[Proved above]
∴ ΔAMC ≅ ΔBMD ...[By SAS congruency]
ii. Since ΔAMC ≅ ΔBMD
∠MAC = ∠MBD ...[By corresponding parts of congruent triangles]
But they form a pair of alternate interior angles.
∴ AC ‖ DB
Now, BC is a transversal which intersects parallel lines AC and DB,
∴ ∠BCA + ∠DBC = 180° ...[Co-interior angles]
But ∠BCA = 90° ...[ΔABC is right angled at C]
∴ 90° + ∠DBC = 180°
⇒ ∠DBC = 90°
iii. Again, ΔAMC ≅ ΔBMD ...[Proved above]
∴ AC = BD ...[By corresponding parts of congruent triangles]
Now, in ΔDBC and ΔACB, we have
BD = CA ...[Proved above]
∠DBC = ∠ACB ...[Each 90°]
BC = CB ...[Common]
∴ ΔDBC ≅ ΔACB ...[By SAS congruency]
iv. As ΔDBC ≅ ΔACB
⇒ DC = AB ...[By corresponding parts of congruent triangles]
But DM = CM ...[Given]
∴ CM = `1/2` DC = `1/2` AB
⇒ CM = `1/2` AB.
APPEARS IN
संबंधित प्रश्न
In the given figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.

If ΔABC and ΔPQR are to be congruent, name one additional pair of corresponding parts. What criterion did you use?
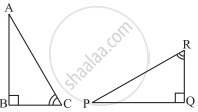
Which of the following statements are true (T) and which are false (F):
If any two sides of a right triangle are respectively equal to two sides of other right triangle, then the two triangles are congruent.
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
In the given figure, prove that:
CD + DA + AB + BC > 2AC
The given figure shows a circle with center O. P is mid-point of chord AB.

Show that OP is perpendicular to AB.
A triangle ABC has ∠B = ∠C.
Prove that: The perpendiculars from the mid-point of BC to AB and AC are equal.
In ∆ABC, AB = AC. Show that the altitude AD is median also.
In the following figure, OA = OC and AB = BC.
Prove that: ΔAOD≅ ΔCOD
In ΔABC, AB = AC and the bisectors of angles B and C intersect at point O.
Prove that : (i) BO = CO
(ii) AO bisects angle BAC.
