Advertisements
Advertisements
प्रश्न
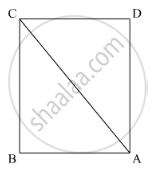
In the following diagram, ABCD is a square and APB is an equilateral triangle.
(i) Prove that: ΔAPD ≅ ΔBPC
(ii) Find the angles of ΔDPC.
उत्तर
Given: ABCD is a Square and ΔAPB is an equilateral triangle.
(i) Proof: In ΔAPB,
AP = PB = AB ...[ APB is an equilateral triangle ]
Also, we have,
∠PBA = ∠PAB = ∠APB = 60° ...(1)
Since ABCD is a square, we have
∠A =∠ B = ∠C = ∠D = 90° ...(2)
Since ∠DAP = ∠A + ∠PAB ..(3)
⇒ ∠DAP = 90° + 60°
⇒ ∠DAP = 150° ...[ from (1) and (2) ] ...(4)
Similarly ∠CBP = ∠B + ∠PBA
⇒ ∠CBP = 90° + 60°
⇒ ∠CBP = 150° ...[ from (1) and (2) ] ...(5)
⇒ ∠DAP = ∠CBP ....[ from (1) and (2) ] ...(6)
In ΔAPD and ΔBPC
AD = BC ...[ Sides of square ABCD ]
∠DAP = ∠CBP ...[ from(6) ]
AP= BP [ Sides of equilateral ΔAPB ]
∴ By Side-AAngel-SIde Criterion of Congruence, we have,
ΔAPD ≅ ΔBPC
(ii)
AP = PB = AB ....[ ΔAPB is an equilateral triangle ] ...(7)
AB = BC = CD = DA ...[ Sides of square ABCD ] ...(8)
From (7) and (8), we have
AP = DA aand PB = BC ... (9)
In ΔAPD,
AP = DA ...[ from (9) ]
∠ADP = ∠APD ...[ Angel opposite to equal sides are equal ] ...(10)
∠ADP + ∠APD+ +∠DAP + 180° ...[ Sum of angel of a triangle = 180° ]
⇒ ∠ADP + ∠ADP + 150° = 180° [ from (3), ∠DAP =150° from (10), ∠ADP = ∠APD ]
⇒ ∠ADP + ∠ADP = 180° - 150°
⇒ 2∠ADP = 30°
⇒ ∠ADP = `30^circ/2`
⇒∠ADP= 15°
We have ∠PDC =∠D - ∠ADP
⇒∠PDC =90° - 15°
⇒∠PDC =75° ...(11)
In ΔBPC,
PB = BC ...[ from (9) ]
∴ ∠PCB =∠ BPC ...[Angel opposite to equal sides are equal ] ... (12)
∠PCB + ∠BPC + ∠CBP = 180° ....[ Sum of angel of a triangle = 180° ]
⇒ ∠PCB + ∠PCB + 30° = 180° ....[ from (5), ∠CBP = 150° from (12) , ∠PCB =∠BPC ]
⇒ 2∠PCB = 180° - 150°
⇒ 2∠PCB = `30^circ/2`
⇒ ∠PCB = 15°
We have ∠PCD = ∠C - ∠PCB
⇒ ∠PCD = 90° - 15°
⇒ ∠PCD = 75° ... (13)
In ΔDPC,
∠PDC = 75°
∠PCD = 75°
∠PCD + ∠PDC + ∠DPC = 180° ...[ Sum of angles of a triangle = 180° ]
⇒ 75° + 75° + ∠DPC = 180°
⇒ ∠DPC = 180° - 150°
⇒ ∠DPC = 30°
∴ Angles of DPC, are: 75°, 30° , 75°
APPEARS IN
संबंधित प्रश्न
You want to show that ΔART ≅ ΔPEN,
If you have to use SSS criterion, then you need to show
1) AR =
2) RT =
3) AT =

In ΔABC, ∠A = 30°, ∠B = 40° and ∠C = 110°
In ΔPQR, ∠P = 30°, ∠Q = 40° and ∠R = 110°
A student says that ΔABC ≅ ΔPQR by AAA congruence criterion. Is he justified? Why or why not?
If perpendiculars from any point within an angle on its arms are congruent, prove that it lies on the bisector of that angle.
Prove that the perimeter of a triangle is greater than the sum of its altitudes.
In the given figure, prove that:
CD + DA + AB + BC > 2AC
If the following pair of the triangle is congruent? state the condition of congruency :
In Δ ABC and Δ DEF, AB = DE, BC = EF and ∠ B = ∠ E.
In a triangle ABC, D is mid-point of BC; AD is produced up to E so that DE = AD.
Prove that :
(i) ΔABD and ΔECD are congruent.
(ii) AB = CE.
(iii) AB is parallel to EC
From the given diagram, in which ABCD is a parallelogram, ABL is a line segment and E is mid-point of BC.
prove that : AL = 2DC
In the following figure, AB = AC and AD is perpendicular to BC. BE bisects angle B and EF is perpendicular to AB.
Prove that: BD = CD

In the adjoining figure, QX and RX are the bisectors of the angles Q and R respectively of the triangle PQR.
If XS ⊥ QR and XT ⊥ PQ ;
prove that: (i) ΔXTQ ≅ ΔXSQ.
(ii) PX bisects angle P.
