Advertisements
Advertisements
प्रश्न
The marks of 200 students in a test is given below :
| Marks% | 10-19 | 20-29 | 30-39 | 40-49 | 50-59 | 60-69 | 70-79 | 80-89 |
| No. of Students | 7 | 11 | 20 | 46 | 57 | 37 | 15 | 7 |
Draw an ogive and find
(i) the median
(ii) the number of students who scored more than 35% marks
उत्तर
We construct cumulative frequency table of the given distribution :
| Marks | No.of students (f) | Cumulative Frequency |
| 9.5-19.5 | 7 | 7 |
| 19.5-29.5 | 11 | 18 |
| 29.5-39.5 | 20 | 38 |
| 39.5-49.5 | 46 | 84 |
| 49.5-59.5 | 57 | 141 |
| 59.5-69.5 | 37 | 178 |
| 69.5-79.5 | 15 | 193 |
| 79.5-89.5 | 7 | 200 |
Take a graph paper and draw both the axes.
On the x -axis , take a scale of 1cm=10 to represent the marks.
On the y - axis , take a scale of 1 cm =50 to represent the no. of students .
Now, plot the points (19.5,7) ,(29.5,18) ,(39.5,38) ,( 49.5,84) ,(59.5,141) ,(69.5,178) ,(79.5,193) ,(89.5,200).
Join them by a smooth curve to get the ogive.
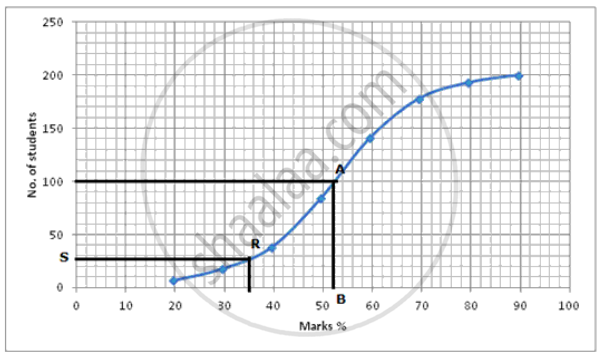
(i) No. of terms = 200
.·. Median= `(100 + 101)/2` = 100.5th term
Through mark of 100.5 on y-axis draw a line parallel to x-axis which meets the curve at A. From A, draw a perpendicular to x-axis which meets it at B.
The value of B is the median which is 52.
(ii) From marks % = 35 draw a line parallel to y-axis and meet the curve at R. From R, Draw a perpendicular on y-axis which meets it at S. The difference of the value obtained when subtracted from 200 gives the number of students who scored more than 35%.
⇒ 200 - 23 = 172
172 students scored more than 35 %
APPEARS IN
संबंधित प्रश्न
Find the mode and the median of the following frequency distribution:
| x | 10 | 11 | 12 | 13 | 14 | 15 |
| f | 1 | 4 | 7 | 5 | 9 | 3 |
The following table gives the age of 50 student of a class. Find the arithmetic mean of their ages.
| Age-years | 16 – 18 | 18 – 20 | 20 – 22 | 22 – 24 | 24 – 26 |
| No. of students | 2 | 7 | 21 | 17 | 3 |
The following table shows the frequency distribution of heights of 51 boys:
| Height (cm) | 120 | 121 | 122 | 123 | 124 |
| Frequency | 5 | 8 | 18 | 10 | 9 |
Find the mode of heights.
The marks of 200 students in a test were recorded as follows:
| Marks | No. of students |
| 10-19 | 7 |
| 20-29 | 11 |
| 30-39 | 20 |
| 40-49 | 46 |
| 50-59 | 57 |
| 60-69 | 37 |
| 70-79 | 15 |
| 80-89 | 7 |
Construct the cumulative frequency table. Drew the ogive and use it too find:
(1) the median and
(2) the number of student who score more than 35% marks.
Q 11
If the mean of 8 , 14 , 20 , x and 12 is 13, find x.
The marks obtained by 200 students in an examination are given below :
| Marks | 0-10 | 10-20 | 20-30 | 30-40 | 40-50 | 50-60 | 60-70 | 70-80 | 80-90 | 90-100 |
| No.of students | 5 | 10 | 11 | 20 | 27 | 38 | 40 | 29 | 14 | 6 |
Using a graph paper, draw an Ogive for the above distribution. Use your Ogive to estimate:
(i) the median;
(ii) the lower quartile;
(iii) the number of students who obtained more than 80% marks in the examination and
(iv) the number of students who did not pass, if the pass percentage was 35.
Use the scale as 2 cm = 10 marks on one axis and 2 cm = 20 students on the other axis.
The mean of 5 numbers is 27. If one new number is included, the new mean is 25. Find the included number.
Find the mean and the median of: 2, 4, 5, 8, 10,13 and 14
The measures of central tendency may not lie between the maximum and minimum values of data.
