Advertisements
Advertisements
प्रश्न
Two ships are there in the sea on either side of a light house in such a way that the ships and the light house are in the same straight line. The angles of depression of two ships as observed from the top of the light house are 60° and 45°. If the height of the light house is 200 m, find the distance between the two ships. [use √3=1.73]
उत्तर
Let d be the distance between the two ships. Suppose the distance of one of the ships from the light house is x metres, then the distance of the other ship from the light house is (d−x) metres.
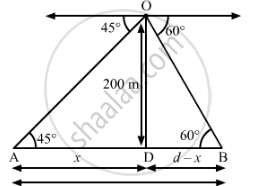
In right-angled ∆ ADO, we have:
`tan 45^@="OD"/"AD"=200/x`
`=>1=200/x`
`=>x=200 ............(1)`
In right-angled ∆ BDO, we have:
`tan60^@="OD"/"BD"=200/(d-x)`
`=>sqrt3=200/(d-x)`
`=>d-x=200/sqrt3`
Putting x= 200, we have:
`d-200=200/sqrt3`
`d=200/sqrt3+200`
`=>d=200((sqrt3+1)/sqrt3)`
`=>d=200xx1.58`
`=>d=316 ` (approx)
Thus, the distance between two ships is approximately 316 m
APPEARS IN
संबंधित प्रश्न
From a point on the ground 40 m away from the foot of a tower, the angle of elevation of the top of the tower is 30º. The angle of elevation of the top of a water tank (on the top of the tower) is 45º. Find the (i) height of the tower (ii) the depth of the tank.
From the top of a 7 m high building, the angle of elevation of the top of a cable tower is 60° and the angle of depression of its foot is 45°. Determine the height of the tower.
An aeroplane flying horizontally 1 km above the ground is observed at an elevation of 60°. After 10 seconds, its elevation is observed to be 30°. Find the speed of the aeroplane in km/hr.
From the top of a lighthouse, the angles of depression of two ships on the opposite sides of it are observed to be a and 3. If the height of the lighthouse be h meters and the line joining the ships passes through the foot of the lighthouse, show that the distance
`(h(tan alpha + tan beta))/(tan alpha tan beta)` meters
A ladder of length 6meters makes an angle of 45° with the floor while leaning against one wall of a room. If the fort of the ladder is kept fixed on the floor and it is made to lean against the opposite wall of the room, it makes an angle of 60° with the floor. Find the
distance between two walls of the room.
The ratio of the length of a rod and its shadow is `1 : sqrt3`. The angle of elevation of the sum is
It is found that on walking x meters towards a chimney in a horizontal line through its base, the elevation of its top changes from 30° to 60°. The height of the chimney is
Two buildings are in front of each other on either side of a road of width 10 metres. From the top of the first building which is 20 metres high, the angle of elevation to the top of the second is 45°. What is the height of the second building?
A bird is flying from A towards B at an angle of 35°, a point 30 km away from A. At B it changes its course of flight and heads towards C on a bearing of 48° and distance 32 km away. How far is B to the North of A?
(sin 55° = 0.8192, cos 55° = 0.5736, sin 42° = 0.6691, cos 42° = 0.7431)
The angle of elevation of the top of a tower is 30°. If the height of the tower is doubled, then the angle of elevation of its top will also be doubled.
