Advertisements
Advertisements
प्रश्न
Two unequal resistances, R1 and R2, are connected across two identical batteries of emf ε and internal resistance r (see the figure). Can the thermal energies developed in R1 and R2 be equal in a given time? If yes, what will be the condition?

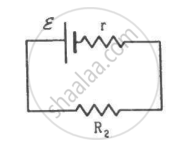
उत्तर
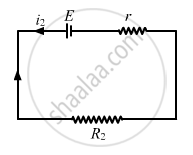
For the given time t, let the currents passing through the resistance R1 and R2 be i1 and i2, respectively.
Applying Kirchoff's Voltage Law to circuit-1, we get:-
\[\epsilon - i_1 r - i_1 R_1 = 0\]
\[ \Rightarrow i_1 = \frac{\epsilon}{r + R_1}\]
Similarly, the current in the other circuit,
\[i_2 = \frac{\epsilon}{r + R_2}\]
The thermal energies through the resistances are given by
\[i_1^2 R_1 t = i_2^2 R_2 t\]
\[ \left( \frac{\epsilon}{r + R_1} \right)^2 R_1 t = \left( \frac{\epsilon}{r + R_2} \right)^2 R_2 t\]
\[\frac{R_1}{\left( r + R_1 \right)^2} = \frac{R_2}{\left( r + R_2 \right)^2}\]
\[\frac{\left( r^2 + {R_1}^2 + 2r R_1 \right)}{R_1} = \frac{\left( r^2 + {R_2}^2 + 2r R_2 \right)}{R_2}\]
\[\frac{r^2}{R_1} + R_1 = \frac{r^2}{R_2} + R_2 \]
\[ r^2 \left( \frac{1}{R_1} - \frac{1}{R_2} \right) = R_2 - R_1 \]
\[ r^2 \times \frac{R_2 - R_1}{R_1 R_2} = R_2 - R_1 \]
\[ r^2 = R_1 R_2 \]
\[ \Rightarrow r = \sqrt{R_1 R_2}\]
APPEARS IN
संबंधित प्रश्न
Kirchhoff's voltage law and current law are respectively in accordance with the conservation of .................................. .
- charge and momentum
- charge and energy
- energy and charge
- energy and momentum
Determine the current in each branch of the network shown in figure.

Given n resistors each of resistance R, how will you combine them to get the (i) maximum (ii) minimum effective resistance? What is the ratio of the maximum to minimum resistance?
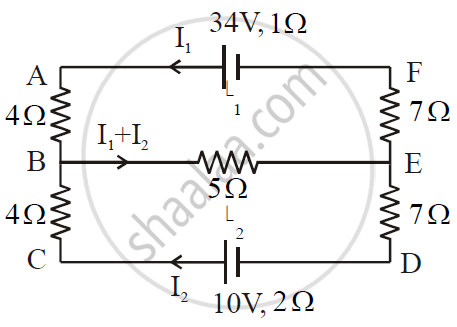
ε1 and ε2 are two batteries having emf of 34V and 10V respectively and internal resistance of 1Ω and 2Ω respectively. They are connected as shown in the figure below. Using Kirchhoff’s Laws of electrical networks, calculate the currents I1 and I2.
Given the resistances of 1 Ω, 2 Ω, 3 Ω, how will be combine them to get an equivalent resistance of (11/3) Ω?
Determine the equivalent resistance of networks shown in Fig.

Find the circuit in the three resistors shown in the figure.
Twelve wires, each of equal resistance r, are joined to form a cube, as shown in the figure. Find the equivalent resistance between the diagonally-opposite points a and f.

A capacitor of capacitance 8.0 μF is connected to a battery of emf 6.0 V through a resistance of 24 Ω. Find the current in the circuit (a) just after the connections are made and (b) one time constant after the connections are made.
On which conservation principle is Kirchoff's Second Law of electrical networks based?
State Kirchhoff ’s voltage rule.
A copper wire of 10-6 m2 area of cross-section, carries a current of 2 A. If the number of electrons per cubic meter is 8 × 1028, calculate the current density and average drift velocity.
A potentiometer wire has a length of 4 m and resistance of 20 Ω. It is connected in series with resistance of 2980 Ω and a cell of emf 4 V. Calculate the potential along the wire.
The Kirchhoff's second law (ΣiR = ΣE), where the symbols have their usual meanings, is based on ______.
Kirchhoff s second law is based on the law of conservation of ______
Why are alloys used for making standard resistance coils?
Two cells of voltage 10V and 2V and internal resistances 10Ω and 5Ω respectively, are connected in parallel with the positive end of 10V battery connected to negative pole of 2V battery (Figure). Find the effective voltage and effective resistance of the combination.
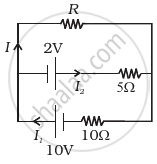
State the two Kirchhoff’s rules used in the analysis of electric circuits and explain them.
Derive the equation of the balanced state in a Wheatstone bridge using Kirchhoff’s laws.
In the circuit shown in Figure below, E1 and E2 are batteries having emfs of 25V and 26V. They have an internal resistance of 1 Ω and 5 Ω respectively. Applying Kirchhoff’s laws of electrical networks, calculate the currents I1 and I2.

