Advertisements
Advertisements
प्रश्न
Verify the existence of `lim_(x -> 1) f(x)`, where `f(x) = {{:((|x - 1|)/(x - 1)",", "for" x ≠ 1),(0",", "for" x = 1):}`
उत्तर
Given `f(x) = {{:((|x - 1|)/(x - 1)",", "for" x ≠ 1),(0",", "for" x = 1):}`
`f(x) = {{:(|(x - 1|)/(x - 1), "for" x < 1 and x > 1),(0, "for" x = 1):}`
`f(x) = {{:((- (x - 1))/(x - 1), "for" x < 1),((x - 1)/(x - 1), "for" x > 1),(0, "for" x = 1):}`
`f(x) = {{:(-1, "for" x < 1),(1, "for" x > 1),(0, "for" x = 1):}`
`f(1^-) = lim_(x -> 1^-) f(x)`
= `lim_(x -> 1^-) (- 1)` = – 1 .......(1)
`f(1^+) = lim_(x -> 1^+) f(x)`
= `lim_(x -> 1^+) (1)` = 1 .......(2)
From equations (1) and (2) we get
f(1–) ≠ f(1+)
∴ The limit of f(x) does not exist.
APPEARS IN
संबंधित प्रश्न
Evaluate the following limit:
If `lim_(x -> 1)[(x^4 - 1)/(x - 1)]` = `lim_(x -> "a")[(x^3 - "a"^3)/(x - "a")]`, find all possible values of a
Evaluate the following limit :
`lim_(x -> 7) [(x^3 - 343)/(sqrt(x) - sqrt(7))]`
Evaluate the following :
`lim_(x -> 0)[x/(|x| + x^2)]`
Evaluate the following :
`lim_(x -> 0) {1/x^12 [1 - cos(x^2/2) - cos(x^4/4) + cos(x^2/2) cos(x^4/4)]}`
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 1) f(x)` where `f(x) = {{:(x^2 + 2",", x ≠ 1),(1",", x = 1):}`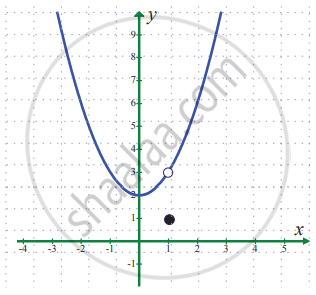
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 3) 1/(x - 3)`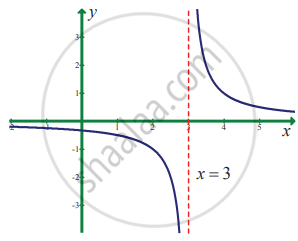
In exercise problems 7 – 15, use the graph to find the limits (if it exists). If the limit does not exist, explain why?
`lim_(x -> 1) sin pi x`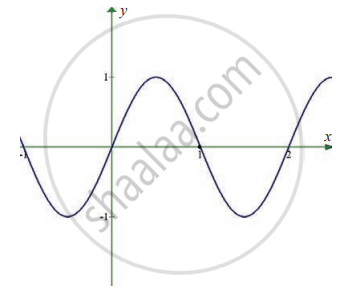
Evaluate the following limits:
`lim_(sqrt(x) -> 3) (x^2 - 81)/(sqrt(x) - 3)`
Evaluate the following limits:
`lim_(x -> 1) (sqrt(x) - x^2)/(1 - sqrt(x))`
Evaluate the following limits:
`lim_(x -> 2) (2 - sqrt(x + 2))/(root(3)(2) - root(3)(4 - x))`
Evaluate the following limits:
`lim_(x -> 5) (sqrt(x - 1) - 2)/(x - 5)`
Evaluate the following limits:
`lim_(x -> 3) (x^2 - 9)/(x^2(x^2 - 6x + 9))`
Evaluate the following limits:
`lim_(x -> oo) (x^3 + x)/(x^4 - 3x^2 + 1)`
Evaluate the following limits:
`lim_(x -> 0) (3^x - 1)/(sqrt(x + 1) - 1)`
Choose the correct alternative:
`lim_(x -> oo) sinx/x`
Choose the correct alternative:
`lim_(x -> 0) sqrt(1 - cos 2x)/x`
Choose the correct alternative:
If `lim_(x -> 0) (sin "p"x)/(tan 3x)` = 4, then the value of p is
Choose the correct alternative:
`lim_(alpha - pi/4) (sin alpha - cos alpha)/(alpha - pi/4)` is
`lim_(x→0^+)(int_0^(x^2)(sinsqrt("t"))"dt")/x^3` is equal to ______.
