Advertisements
Advertisements
प्रश्न
Without using Pythagoras theorem show that points A(4, 4), B(3, 5) and C(−1, −1) are the vertices of a right angled triangle.
उत्तर
Given, A(4, 4), B(3, 5), C(−1, −1).
Slope of AB = `(y_2 - y_1)/(x_2 - x_1) = (5 - 4)/(3 - 4)` = −1
Slope of BC = `(-1 - 5)/(-1 - 3) = (-6)/(-4) = 3/2`
Slope of AC = `(-1 - 4)/(-1 - 4)` = 1
Slope of AB x slope of AC = −1 × 1 = −1
∴ side AB ⊥ side AC
Thus ΔABC is a right angled triangle right angled at A.
∴ The given points are the vertices of a right angled triangle.
APPEARS IN
संबंधित प्रश्न
Find the slope of the following line which passes through the points:
A(2, −1), B(4, 3)
Find the slope of the following line which passes through the points:
C(−2, 3), D(5, 7)
Find the slope of the line which makes angle of 45° with the positive direction of the Y-axis measured anticlockwise
Find the acute angle between the X-axis and the line joining points A(3, −1) and B(4, −2).
If points A(h, 0), B(0, k) and C(a, b) lie on a line then show that `"a"/"h" + "b"/"k"` = 1
Select the correct option from the given alternatives:
If A is (5, −3) and B is a point on the x-axis such that the slope of line AB is −2 then B ≡
Select the correct option from the given alternatives:
If kx + 2y − 1 = 0 and 6x − 4y + 2 = 0 are identical lines, then determine k
Answer the following question:
Find the value of k if the slope of the line passing through the points P(3, 4), Q(5, k) is 9
Find the equation of the lines passing through the point (1, 1) with y-intercept (– 4)
Find the equation of the lines passing through the point (1,1) and (– 2, 3)
Find the equation of the lines passing through the point (1, 1) and the perpendicular from the origin makes an angle 60° with x-axis
Find the equation of the line passing through the point (1, 5) and also divides the co-ordinate axes in the ratio 3:10
The normal boiling point of water is 100°C or 212°F and the freezing point of water is 0°C or 32°F. Find the linear relationship between C and F
An object was launched from a place P in constant speed to hit a target. At the 15th second, it was 1400 m from the target, and at the 18th second 800 m away. Find the distance covered by it in 15 seconds
An object was launched from a place P in constant speed to hit a target. At the 15th second, it was 1400 m from the target, and at the 18th second 800 m away. Find time taken to hit the target
Population of a city in the years 2005 and 2010 are 1,35,000 and 1,45,000 respectively. Find the approximate population in the year 2015. (assuming that the growth of population is constant)
Find the equation of the straight lines passing through (8, 3) and having intercepts whose sum is 1
Show that the points (1, 3), (2, 1) and `(1/2, 4)` are collinear, by using concept of slope
Show that the points (1, 3), (2, 1) and `(1/2, 4)` are collinear, by using any other method
A 150 m long train is moving with constant velocity of 12.5 m/s. Find time taken to cross a pole
A spring was hung from a hook in the ceiling. A number of different weights were attached to the spring to make it stretch, and the total length of the spring was measured each time is shown in the following table
| Weight (kg) | 2 | 4 | 5 | 8 |
| Length (cm) | 3 | 4 | 4.5 | 6 |
Find the equation relating the length of the spring to the weight on it
A spring was hung from a hook in the ceiling. A number of different weights were attached to the spring to make it stretch, and the total length of the spring was measured each time is shown in the following table
| Weight (kg) | 2 | 4 | 5 | 8 |
| Length (cm) | 3 | 4 | 4.5 | 6 |
If the spring has to stretch to 9 cm long, how much weight should be added?
A spring was hung from a hook in the ceiling. A number of different weights were attached to the spring to make it stretch, and the total length of the spring was measured each time is shown in the following table
| Weight (kg) | 2 | 4 | 5 | 8 |
| Length (cm) | 3 | 4 | 4.5 | 6 |
How long will the spring be when 6 kilograms of weight on it?
In a shopping mall there is a hall of cuboid shape with dimension 800 × 800 × 720 units, which needs to be added the facility of an escalator in the path as shown by the dotted line in the figure. Find the slopes of the escalator at the turning points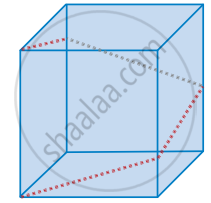
Choose the correct alternative:
Straight line joining the points (2, 3) and (−1, 4) passes through the point (α, β) if
Choose the correct alternative:
Equation of the straight line perpendicular to the line x − y + 5 = 0, through the point of intersection the y-axis and the given line
Choose the correct alternative:
The line (p + 2q)x + (p − 3q)y = p − q for different values of p and q passes through the point
Choose the correct alternative:
The y-intercept of the straight line passing through (1, 3) and perpendicular to 2x − 3y + 1 = 0 is
The distance of the origin from the centroid of the triangle whose two sides have the equations. x – 2y + 1 = 0 and 2x – y – 1 = 0 and whose orthocenter is `(7/3. 7/3)` is ______.
A point on the straight line, 3x + 5y = 15 which is equidistant from the coordinate, axes will lie only in ______.
If planes x – cy – bz = 0, cx – y + az = 0 and bx + ay – z = 0 pass through a straight line then a2 + b2 + c2 = ______.
Find the transformed equation of the straight line 2x – 3y + 5 = 0, when the origin is shifted to the point (3, –1) after translation of axes.
