Advertisements
Advertisements
प्रश्न
A graph representing the function f(x) is given in it is clear that f(9) = 2
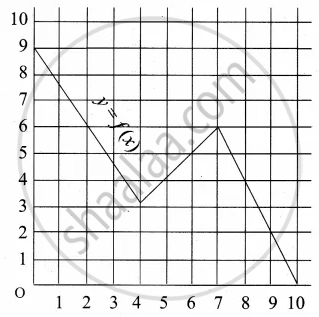
For what value of x is f(x) = 1?
उत्तर
When f(x) = 1 the value of x is 9.5
APPEARS IN
संबंधित प्रश्न
If \[f\left( x \right) = \frac{x + 1}{x - 1}\] , show that f[f[(x)]] = x.
If \[f\left( x \right) = \frac{2^x + 2^{- x}}{2}\] , then f(x + y) f(x − y) is equal to
Let A = {x ∈ R : x ≠ 0, −4 ≤ x ≤ 4} and f : A ∈ R be defined by \[f\left( x \right) = \frac{\left| x \right|}{x}\] for x ∈ A. Then th (is
If f(x) = 3x + a and f(1) = 7 find a and f(4).
Find the domain and range of the follwoing function.
h(x) = `sqrt(x + 5)/(5 + x)`
Find the domain of f(x) = ln (x − 5)
The function f and g are defined by f(x) = 6x + 8; g(x) = `(x - 2)/3`
Calculate the value of `"gg" (1/2)`
The domain of the function f defined by f(x) = `1/sqrt(x - |x|)` is ______.
Find the domain and range of the function f(x) = `1/sqrt(x - 5)`
The domain and range of the function f given by f(x) = 2 – |x – 5| is ______.
