Advertisements
Advertisements
प्रश्न
A graph representing the function f(x) is given in it is clear that f(9) = 2
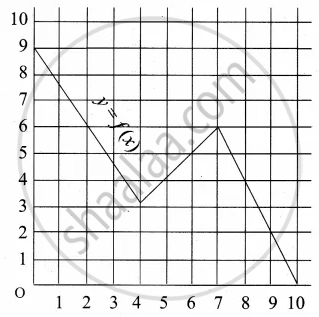
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
उत्तर
(a) f(0) = 9
(b) f(7) = 6
(c) f(2) = 6
(d) f(10) = 0
APPEARS IN
संबंधित प्रश्न
Write the domain and range of function f(x) given by
If \[f\left( x \right) = 64 x^3 + \frac{1}{x^3}\] and α, β are the roots of \[4x + \frac{1}{x} = 3\] . Then,
The domain of definition of the function \[f\left( x \right) = \sqrt{\frac{x - 2}{x + 2}} + \sqrt{\frac{1 - x}{1 + x}}\] is
Check if the relation given by the equation represents y as function of x:
x + y2 = 9
Find the domain and range of the follwoing function.
h(x) = `sqrt(x + 5)/(5 + x)`
Show that if f : A → B and g : B → C are onto, then g ° f is also onto
Given the function f: x → x2 – 5x + 6, evaluate f(2)
Let A = {1, 2, 3, 4} and B = N. Let f : A → B be defined by f(x) = x3 then, find the range of f
If f(x) = y = `(ax - b)/(cx - a)`, then prove that f(y) = x.
The ratio `(2^(log_2 1/4 a) - 3^(log_27(a^2 + 1)^3) - 2a)/(7^(4log_49a) - a - 1)` simplifies to ______.
