Advertisements
Advertisements
प्रश्न
A graph representing the function f(x) is given in it is clear that f(9) = 2
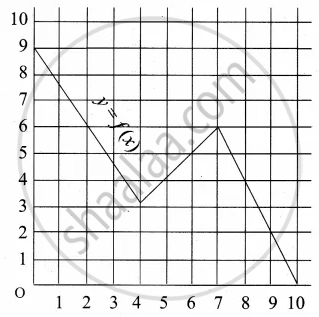
Find the following values of the function
(a) f(0)
(b) f(7)
(c) f(2)
(d) f(10)
उत्तर
(a) f(0) = 9
(b) f(7) = 6
(c) f(2) = 6
(d) f(10) = 0
APPEARS IN
संबंधित प्रश्न
Let f(x) = x2 and g(x) = 2x+ 1 be two real functions. Find (f + g) (x), (f − g) (x), (fg) (x) and \[\left( \frac{f}{g} \right) \left( x \right)\] .
Write the range of the function f(x) = ex−[x], x ∈ R.
Express the following exponential equation in logarithmic form
25 = 32
Express the following exponential equation in logarithmic form
54° = 1
Select the correct answer from given alternatives.
If log (5x – 9) – log (x + 3) = log 2 then x = ...............
Answer the following:
Identify the following relation is the function? If it is a function determine its domain and range.
{(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
The function f and g are defined by f(x) = 6x + 8; g(x) = `(x - 2)/3`
Write an expression for gf(x) in its simplest form
Let A and B be any two sets such that n(B) = p, n(A) = q then the total number of functions f : A → B is equal to ______.
Range of f(x) = `1/(1 - 2 cosx)` is ______.
The domain and range of the real function f defined by f(x) = `(4 - x)/(x - 4)` is given by ______.
