Advertisements
Advertisements
प्रश्न
The domain of the function f defined by f(x) = `1/sqrt(x - |x|)` is ______.
पर्याय
R
R+
R–
None of these
उत्तर
none of these
Explanation:
Given that f(x) = `1/sqrt(x - |x|)`
Where `x - |x| = {{:(x - x = 0, "if" x ≥ 0),(2x, "if" x < 0):}`
Thus `1/sqrt(x - |x|)` is not defined for any x ∈ R.
Hence f is not defined for any x ∈ R.
APPEARS IN
संबंधित प्रश्न
If \[f\left( x \right) = \frac{x - 1}{x + 1}\] , then show that
(i) \[f\left( \frac{1}{x} \right) = - f\left( x \right)\]
(ii) \[f\left( - \frac{1}{x} \right) = - \frac{1}{f\left( x \right)}\]
If f(x) = cos [π2]x + cos [−π2] x, where [x] denotes the greatest integer less than or equal to x, then write the value of f(π).
Write the range of the function f(x) = ex−[x], x ∈ R.
Write the domain and range of function f(x) given by \[f\left( x \right) = \sqrt{\left[ x \right] - x}\] .
Let A = {1, 2, 3} and B = {2, 3, 4}. Then which of the following is a function from A to B?
If A = {1, 2, 3} and B = {x, y}, then the number of functions that can be defined from A into B is
The domain of definition of the function \[f\left( x \right) = \sqrt{\frac{x - 2}{x + 2}} + \sqrt{\frac{1 - x}{1 + x}}\] is
The domain of definition of \[f\left( x \right) = \sqrt{x - 3 - 2\sqrt{x - 4}} - \sqrt{x - 3 + 2\sqrt{x - 4}}\] is
The range of \[f\left( x \right) = \frac{1}{1 - 2\cos x}\] is
Check if the following relation is function:
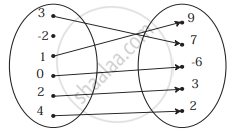
Find the domain and range of the following function.
g(x) = `(x + 4)/(x - 2)`
Express the area A of circle as a function of its radius r
Show that if f : A → B and g : B → C are one-one, then g ° f is also one-one
Express the following exponential equation in logarithmic form
10−2 = 0.01
Express the following exponential equation in logarithmic form
e2 = 7.3890
The equation logx2 16 + log2x 64 = 3 has,
Answer the following:
Identify the following relation is the function? If it is a function determine its domain and range
{(12, 1), (3, 1), (5, 2)}
Answer the following:
Find whether the following function is one-one
f : R − {3} → R defined by f(x) = `(5x + 7)/(x - 3)` for x ∈ R − {3}
Answer the following:
Let f : R – {2} → R be defined by f(x) = `(x^2 - 4)/(x - 2)` and g : R → R be defined by g(x) = x + 2. Examine whether f = g or not
Answer the following:
If `log"a"/(x + y - 2z) = log"b"/(y + z - 2x) = log"c"/(z + x - 2y)`, show that abc = 1
Answer the following:
Find the domain of the following function.
f(x) = x!
A function f is defined by f(x) = 2x – 3 find `("f"(0) + "f"(1))/2`
Let A = {1, 2, 3, 4} and B = N. Let f : A → B be defined by f(x) = x3 then, find the range of f
The range of 7, 11, 16, 27, 31, 33, 42, 49 is ______.
The range of the function f(x) = `(x - 3)/(5 - x)`, x ≠ 5 is ______.
Domain of function f(x) = cos–1 6x is ______.
Let A and B be any two sets such that n(B) = p, n(A) = q then the total number of functions f : A → B is equal to ______.
Find the domain of the following function given by:
f(x) = `(3x)/(2x - 8)`
Range of f(x) = `1/(1 - 2 cosx)` is ______.
