Advertisements
Advertisements
प्रश्न
A motorcycle has to move with a constant speed on an over bridge which is in the form of a circular arc of radius R and has a total length L. Suppose the motorcycle starts from the highest point.(a) What can its maximum velocity be for which the contact with the road is not broken at the highest point? (b) If the motorcycle goes at speed 1/√2 times the maximum found in part (a), where will it lose the contact with the road? (c) What maximum uniform speed can it maintain on the bridge if it does not lose contact anywhere on the bridge?
उत्तर
R = Radius of the bridge
L = Total length of the over bridge
(a) At the highest point:
Let m be the mass of the motorcycle and v be the required velocity.
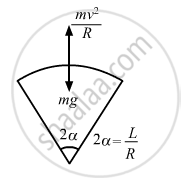
\[\text{mg }= \frac{\text{mv}^2}{\text{R}}\]
\[ \Rightarrow \text{v}^2 = \text{Rg}\]
\[ \Rightarrow \text{v} = \sqrt{\text{Rrg}}\]
\[\left( b \right) \text {Given :} \]
\[ \text{v} = \left( \frac{1}{\sqrt{2}} \right)\sqrt{\text{Rg}}\]

Suppose it loses contact at B.
\[\text {At point B, we get : }\]
\[\text{mg}\cos\theta = \frac{\text{mv}^2}{R}\]
\[ \Rightarrow \text{v}^2 = \text{Rg}\cos\theta\]
\[\text {Putting the value of v}, \text {we get : } \]
\[\sqrt{\left( \frac{Rg}{2} \right)^2} = Rg\cos\theta\]
\[ \Rightarrow \frac{Rg}{2} = Rg\cos\theta\]
\[ \Rightarrow \cos\theta = \frac{1}{2}\]
\[ \Rightarrow \theta = 60^\circ = \frac{\pi}{3}\]
\[ \because \theta = \frac{L}{R}\]
\[ \therefore L = R\theta = \frac{\pi R}{3}\]
So, it will lose contact at a distance \[\frac{\pi R}{3}\] from the highest point.
(c) Let the uniform speed on the bridge be v. The chances of losing contact is maximum at the end bridge. We have :
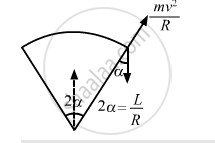
\[\alpha = \frac{L}{2R}\]
\[\text{So}, \frac{\text{mv}^2}{R} = \text{mg}\cos\alpha\]
\[ \Rightarrow v = \sqrt{\text{gRcos}\left( \frac{L}{2R} \right)}\]
APPEARS IN
संबंधित प्रश्न
A particle is kept fixed on a turntable rotating uniformly. As seen from the ground the particle goes in a circle, its speed is 20 cm/s and acceleration is 20 cm/s2. The particle is now shifted to a new position to make the radius half of the original value. The new value of the speed and acceleration will be
Water in a bucket is whirled in a vertical circle with string attached to it. The water does no fall down even when the bucket is inverted at the top of its path. We conclude that in this position
A train A runs from east to west and another train B of the same mass runs from west to east at the same speed along the equator. A presses the track with a force F1 and B presses the track with a force F2.
Assume that the earth goes round the sun in a circular orbit with a constant speed of 30 kms
Find the acceleration of a particle placed on the surface of the earth at the equator due to earth's rotation. The diameter of earth = 12800 km and it takes 24 hours for the earth to complete one revolution about its axis.
The bob of a simple pendulum of length 1 m has mass 100 g and a speed of 1.4 m/s at the lowest point in its path. Find the tension in the string at this instant.
A person stands on a spring balance at the equator. By what fraction is the balance reading less than his true weight?
A hemispherical bowl of radius R is rotated about its axis of symmetry which is kept vertical. A small block is kept in the bowl at a position where the radius makes an angle θ with the vertical. The block rotates with the bowl without any slipping. The friction coefficient between the block and the bowl surface is μ. Find the range of the angular speed for which the block will not slip.
A particle is projected with a speed u at an angle θ with the horizontal. Consider a small part of its path near the highest position and take it approximately to be a circular arc. What is the radius of this circular circle? This radius is called the radius of curvature of the curve at the point.
A particle of mass 1 kg, tied to a 1.2 m long string is whirled to perform the vertical circular motion, under gravity. The minimum speed of a particle is 5 m/s. Consider the following statements.
P) Maximum speed must be `5sqrt5` m/s.
Q) Difference between maximum and minimum tensions along the string is 60 N.
Select the correct option.
A body slides down a smooth inclined plane having angle θ and reaches the bottom with velocity v. If a body is a sphere, then its linear velocity at the bottom of the plane is
Two particles A and B are located at distances rA and rB respectively from the centre of a rotating disc such that rA > rB. In this case, if angular velocity ω of rotation is constant, then ______
The centripetal force of a body moving in a circular path, if speed is made half and radius is made four times the original value, will ____________.
Angular displacement (θ) of a flywheel varies with time as θ = at + bt2 + ct3 then angular acceleration is given by ____________.
If a cyclist doubles his speed while negotiating a curve, how does the tendency to overturn vary?
A body is moving along a circular track of radius 100 m with velocity 20 m/s. Its tangential acceleration is 3 m/s2 then its resultant accelaration will be ______.
When a body slides down from rest along a smooth inclined plane making an angle of 45° with the horizontal, it takes time T. When the same body slides down from rest along a rough inclined plane making the same angle and through the same distance, it is seen to take time pT, where p is some number greater than 1. Calculate the co-efficient of friction between the body and the rough plane.
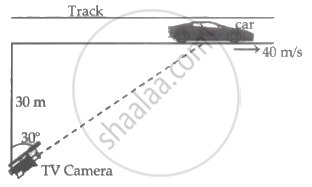
A racing car is travelling along a track at a constant speed of 40 m/s. A T.V. cameraman is recording the event from a distance of 30 m directly away from the track as shown in the figure. In order to keep the car under view in the position shown, the angular speed with which the camera should be rotated is ______.