Advertisements
Advertisements
प्रश्न
A simple pendulum of length l is suspended through the ceiling of an elevator. Find the time period of small oscillations if the elevator (a) is going up with and acceleration a0(b) is going down with an acceleration a0 and (c) is moving with a uniform velocity.
उत्तर
The length of the simple pendulum is l.
Let x be the displacement of the simple pendulum..
(a)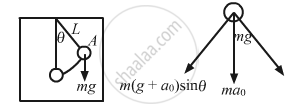
From the diagram, the driving forces f is given by,
f = m(g + a0)sinθ ...(1)
Acceleration (a) of the elevator is given by,
\[a = \frac{f}{m}\]
\[ = \left( g + a_0 \right)\sin\theta\]
\[ = \left( g + a_0 \right)\frac{x}{l} \left( \text{From the diagram } \sin\theta = \frac{x}{l} \right)_{}\]
[ when θ is very small, sin θ → θ = x/l]
\[\therefore a = \left( \frac{g + a_0}{l} \right)x\] ...(2)
As the acceleration is directly proportional to displacement, the pendulum executes S.H.M.
Comparing equation (2) with the expression a =\[\omega^2 x\],we get:
\[\omega^2 = \frac{g + a_0}{l}\]
Thus, time period of small oscillations when elevator is going upward(T) will be:
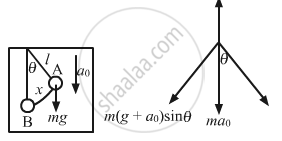
Driving force (F) is given by,
F = m(g − a0)sinθ
On comparing the above equation with the expression, F = ma,\[\text{Acceleration}, a = \left( g - a_0 \right) sin\theta = \frac{\left( g - a_0 \right)x}{l} = \omega^2 x\] \[\text{Time period of elevator when it is moving downward}\left( T' \right) \text{ is given by,} \]
\[T' = \frac{2\pi}{\omega} = 2\pi\sqrt{\frac{l}{g - a_0}}\]
(c) When the elevator moves with uniform velocity, i.e. a0 = 0,
For a simple pendulum, the driving force \[\left( F \right)\] is given by,
\[F = \frac{mgx}{l}\]
\[\text{Comparing the above equation with the expression, F = ma, we get: }\] \[a = \frac{gx}{l}\] \[ \Rightarrow \frac{x}{a} = \frac{l}{g}\] \[T = 2\pi\sqrt{\frac{\text{displacement}}{\text{Acceleration}}}\] \[ = 2\pi\sqrt{\frac{l}{g}}\]
APPEARS IN
संबंधित प्रश्न
A particle in S.H.M. has a period of 2 seconds and amplitude of 10 cm. Calculate the acceleration when it is at 4 cm from its positive extreme position.
In a damped harmonic oscillator, periodic oscillations have _______ amplitude.
(A) gradually increasing
(B) suddenly increasing
(C) suddenly decreasing
(D) gradually decreasing
Can simple harmonic motion take place in a non-inertial frame? If yes, should the ratio of the force applied with the displacement be constant?
A small creature moves with constant speed in a vertical circle on a bright day. Does its shadow formed by the sun on a horizontal plane move in a sample harmonic motion?
The time period of a particle in simple harmonic motion is equal to the smallest time between the particle acquiring a particular velocity \[\vec{v}\] . The value of v is
The displacement of a particle in simple harmonic motion in one time period is
Figure represents two simple harmonic motions.
The parameter which has different values in the two motions is

The average energy in one time period in simple harmonic motion is
The motion of a torsional pendulum is
(a) periodic
(b) oscillatory
(c) simple harmonic
(d) angular simple harmonic
A particle moves in the X-Y plane according to the equation \[\overrightarrow{r} = \left( \overrightarrow{i} + 2 \overrightarrow{j} \right)A\cos\omega t .\]
The motion of the particle is
(a) on a straight line
(b) on an ellipse
(c) periodic
(d) simple harmonic
A particle executes simple harmonic motion with an amplitude of 10 cm and time period 6 s. At t = 0 it is at position x = 5 cm going towards positive x-direction. Write the equation for the displacement x at time t. Find the magnitude of the acceleration of the particle at t = 4 s.
A spherical ball of mass m and radius r rolls without slipping on a rough concave surface of large radius R. It makes small oscillations about the lowest point. Find the time period.
If the inertial mass and gravitational mass of the simple pendulum of length l are not equal, then the time period of the simple pendulum is
Consider the Earth as a homogeneous sphere of radius R and a straight hole is bored in it through its centre. Show that a particle dropped into the hole will execute a simple harmonic motion such that its time period is
T = `2π sqrt("R"/"g")`
Consider a simple pendulum of length l = 0.9 m which is properly placed on a trolley rolling down on a inclined plane which is at θ = 45° with the horizontal. Assuming that the inclined plane is frictionless, calculate the time period of oscillation of the simple pendulum.
A body oscillates with SHM according to the equation x = 5 cos `(2π"t" + π/4)`. Its instantaneous displacement at t = 1 sec is:
The displacement of a particle is represented by the equation y = sin3ωt. The motion is ______.
Motion of a ball bearing inside a smooth curved bowl, when released from a point slightly above the lower point is ______.
- simple harmonic motion.
- non-periodic motion.
- periodic motion.
- periodic but not S.H.M.
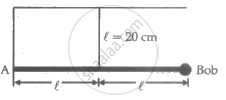
A weightless rigid rod with a small iron bob at the end is hinged at point A to the wall so that it can rotate in all directions. The rod is kept in the horizontal position by a vertical inextensible string of length 20 cm, fixed at its midpoint. The bob is displaced slightly, perpendicular to the plane of the rod and string. The period of small oscillations of the system in the form `(pix)/10` is ______ sec. and the value of x is ______.
(g = 10 m/s2)