Advertisements
Advertisements
प्रश्न
A small source of sound oscillates in simple harmonic motion with an amplitude of 17 cm. A detector is placed along the line of motion of the source. The source emits a sound of frequency 800 Hz which travels at a speed of 340 m s−1. If the width of the frequency band detected by the detector is 8 Hz, find the time period of the source.
उत्तर
Given:
Amplitude r = 17 cm = \[\frac{17}{100}\]= 0.17 m
Frequency of sound emitted by source f = 800 Hz
Velocity of sound \[v\]= 340 m/s
Frequency band = f2\[-\]f1= 8 Hz
Here,
\[f_2\] and \[f_1\]correspond to the maximum and minimum apparent frequencies (Both will be at the mean position because the velocity is maximum).
\[\text { Now }, f_1 = \left( \frac{340}{340 + v_s} \right)f\]
\[ \text { and } f_2 = \left( \frac{340}{340 - v_s} \right)f\]
\[ \therefore f_2 - f_1 = 8\]
\[ \Rightarrow 8 = \left( \frac{340}{340 - v_s} \right)f - \left( \frac{340}{340 + v_s} \right)f\]
\[ \Rightarrow 8 = 340f\left[ \frac{1}{340 - v_s} - \frac{1}{340 + v_s} \right]\]
\[ \Rightarrow 8 = 340 \times 800 \times \left[ \frac{2 v_s}{{340}^2 - {v_s}^2} \right]\]
\[ \Rightarrow \frac{2 v_s}{{340}^2 - v_s^2} = \frac{8}{340 \times 800}\]
\[ \Rightarrow {340}^2 - {v_s}^2 = 68000 v_s \]
Solving for vs, we get:
\[v_s\]= 1.695 m/s
For SHM:
\[v_s = r\omega\]
\[ \Rightarrow \omega = \left( \frac{1 . 695}{0 . 17} \right) = 10\]
\[ \therefore T = \frac{2\pi}{w} = \frac{\pi}{5} = 0 . 63 \sec\]
APPEARS IN
संबंधित प्रश्न
A wave is represented by an equation \[y = c_1 \sin \left( c_2 x + c_3 t \right)\] In which direction is the wave going? Assume that \[c_1 , c_2\] \[c_3\] are all positive.
A string clamped at both ends vibrates in its fundamental mode. Is there any position (except the ends) on the string which can be touched without disturbing the motion? What if the string vibrates in its first overtone?
Can you hear your own words if you are standing in a perfect vacuum? Can you hear your friend in the same conditions?
Two tuning forks vibrate with the same amplitude but the frequency of the first is double the frequency of the second. Which fork produces more intense sound in air?
When we clap our hands, the sound produced is best described by Here p denotes the change in pressure from the equilibrium value.
An electrically maintained tuning fork vibrates with constant frequency and constant amplitude. If the temperature of the surrounding air increases but pressure remains constant, the produced will have
(a) larger wavelength
(b) larger frequency
(c) larger velocity
(d) larger time period.
A source of sound moves towards an observer.
A man stands before a large wall at a distance of 50.0 m and claps his hands at regular intervals. Initially, the interval is large. He gradually reduces the interval and fixes it at a value when the echo of a clap merges every 3 seconds, find the velocity of sound in air.
A string, fixed at both ends, vibrates in a resonant mode with a separation of 2⋅0 cm between the consecutive nodes. For the next higher resonant frequency, this separation is reduced to 1⋅6 cm. Find the length of the string.
A particular guitar wire is 30⋅0 cm long and vibrates at a frequency of 196 Hz when no finger is placed on it. The next higher notes on the scale are 220 Hz, 247 Hz, 262 Hz and 294 Hz. How far from the end of the string must the finger be placed to play these notes?
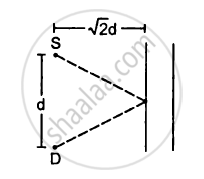
A source S and a detector D are placed at a distance d apart. A big cardboard is placed at a distance \[\sqrt{2}d\] from the source and the detector as shown in figure. The source emits a wave of wavelength = d/2 which is received by the detector after reflection from the cardboard. It is found to be in phase with the direct wave received from the source. By what minimum distance should the cardboard be shifted away so that the reflected wave becomes out of phase with the direct wave?
A source of sound with adjustable frequency produces 2 beats per second with a tuning fork when its frequency is either 476 Hz of 480 Hz. What is the frequency of the tuning fork?
Show that if the room temperature changes by a small amount from T to T + ∆T, the fundamental frequency of an organ pipe changes from v to v + ∆v, where \[\frac{∆ v}{v} = \frac{1}{2}\frac{∆ T}{T} .\]
A boy riding on a bicycle going at 12 km h−1 towards a vertical wall whistles at his dog on the ground. If the frequency of the whistle is 1600 Hz and the speed of sound in air is 330 m s−1, find (a) the frequency of the whistle as received by the wall (b) the frequency of the reflected whistle as received by the boy.
A car moves with a speed of 54 km h−1 towards a cliff. The horn of the car emits sound of frequency 400 Hz at a speed of 335 m s−1. (a) Find the wavelength of the sound emitted by the horn in front of the car. (b) Find the wavelength of the wave reflected from the cliff. (c) What frequency does a person sitting in the car hear for the reflected sound wave? (d) How many beats does he hear in 10 seconds between the sound coming directly from the horn and that coming after the reflection?
Equation of a plane progressive wave is given by `y = 0.6 sin 2π (t - x/2)`. On reflection from a denser medium its amplitude becomes 2/3 of the amplitude of the incident wave. The equation of the reflected wave is ______.
In an experiment to determine the velocity of sound in air at room temperature using a resonance tube, the first resonance is observed when the air column has a length of 20.0 cm for a tuning fork of frequency 400 Hz is used. The velocity of the sound at room temperature is 336 ms-1. The third resonance is observed when the air column has a length of ______ cm.
The speed of a wave in a string is 20 m/s and the frequency is 50 Hz. The phase difference between two points on the string 10 cm apart will be ______.
