Advertisements
Advertisements
प्रश्न
A tunnel is dug along a diameter of the earth. Find the force on a particle of mass m placed in the tunnel at a distance x from the centre.
उत्तर
Mass of the Earth,
\[M = \left( \frac{4}{3} \right)\pi R^3 \rho\] ...(i)
Consider an imaginary sphere of radius x with centre O as shown in the figure below :
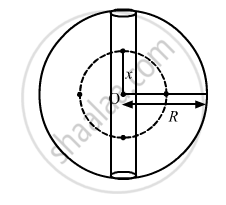
\[\text { Mass of the imaginary sphere }, M' = \left( \frac{4}{3} \right)\pi x^3 \rho . . . (ii)\]
\[\text { From (i) and (ii), we have : }\]
\[\frac{M'}{M} = \frac{x^3}{R^3}\]
∴ Gravitational force on the particle of mass m is given by F \[= \frac{GMm}{x^2}\]
\[\Rightarrow F = \frac{GM x^3 m}{R^3 x^2} = \frac{GMm}{R^3}x\]
APPEARS IN
संबंधित प्रश्न
State and explain Kepler's laws of planetary motion. Draw diagrams to illustrate these laws.
At noon, the sun and the earth pull the objects on the earth's surface in opposite directions. At midnight, the sun and the earth pull these objects in same direction. Is the weight of an object, as measured by a spring balance on the earth's surface, more at midnight as compared to its weight at noon?
Inside a uniform spherical shell
(a) the gravitational potential is zero
(b) the gravitational field is zero
(c) the gravitational potential is same everywhere
(d) the gravitational field is same everywhere
Consider a planet moving in an elliptical orbit round the sun. The work done on the planet by the gravitational force of the sun
(a) is zero in any small part of the orbit
(b) is zero in some parts of the orbit
(c) is zero in one complete revolution
(d) is zero in no part of the motion.
Four particles having masses m, 2m, 3m and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the centre.
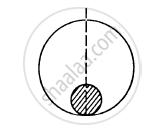
A solid sphere of mass m and radius r is placed inside a hollow thin spherical shell of mass M and radius R as shown in the following figure . A particle of mass m' is placed on the line joining the two centres at a distance x from the point of contact of the sphere and the shell. Find the magnitude of the resultant gravitational force on this particle due to the sphere and the shell if (a) r < x < 2r, (b) 2r < x < 2R and (c) x > 2R.
The law of gravitation gives the gravitational force between :
Explain the following:
People often shake the branches of a tree for getting down its fruits.
Who stated the law of gravitation?
How will the force of gravitation between two objects change if the distance between them is:
Almost zero
What does a force do in the following case?
You apply brakes to a running car.
Name and state the action and reaction in the following case:
Hammering a nail.
Two equal and opposite forces acting at the same point on a stationary body. Will the body move? Give reason to explain your answer.
Explain why:
The atmosphere does not escape.
Solve the following problem.
Find the gravitational force between the Sun and the Earth.
Given Mass of the Sun = 1.99 × 1030 kg
Mass of the Earth = 5.98 × 1024 kg
The average distance between the Earth and the Sun = 1.5 × 1011 m.
The _______ force is much weaker than other forces in nature.
To project the rockets which of the following principle(s) is /(are) required?
Three uniform spheres, each having mass m and radius r, are kept in such a way that each touches the other two. The magnitude of the gravitational force on any sphere due to the other two is
We can shield a charge from electric fields by putting it inside a hollow conductor. Can we shield a body from the gravitational influence of nearby matter by putting it inside a hollow sphere or by some other means?
Six point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.
