Advertisements
Advertisements
Question
A tunnel is dug along a diameter of the earth. Find the force on a particle of mass m placed in the tunnel at a distance x from the centre.
Solution
Mass of the Earth,
\[M = \left( \frac{4}{3} \right)\pi R^3 \rho\] ...(i)
Consider an imaginary sphere of radius x with centre O as shown in the figure below :
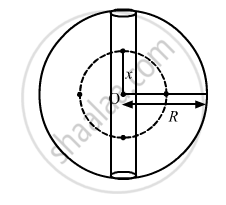
\[\text { Mass of the imaginary sphere }, M' = \left( \frac{4}{3} \right)\pi x^3 \rho . . . (ii)\]
\[\text { From (i) and (ii), we have : }\]
\[\frac{M'}{M} = \frac{x^3}{R^3}\]
∴ Gravitational force on the particle of mass m is given by F \[= \frac{GMm}{x^2}\]
\[\Rightarrow F = \frac{GM x^3 m}{R^3 x^2} = \frac{GMm}{R^3}x\]
APPEARS IN
RELATED QUESTIONS
Suppose the gravitational potential due to a small system is k/r2 at a distance r from it. What will be the gravitational field? Can you think of any such system? What happens if there were negative masses?
A person brings a mass of 1 kg from infinity to a point A. Initially the mass was at rest but it moves at a speed of 2 m s −1 as it reaches A. The work done by the person on the mass is −3 J. The potential at A is
A body is suspended from a spring balance kept in a satellite. The reading of the balance is W1 when the satellite goes in an orbit of radius R and is W2 when it goes in an orbit of radius 2 −R.
Inside a uniform spherical shell
(a) the gravitational potential is zero
(b) the gravitational field is zero
(c) the gravitational potential is same everywhere
(d) the gravitational field is same everywhere
Four particles having masses m, 2m, 3m and 4m are placed at the four corners of a square of edge a. Find the gravitational force acting on a particle of mass m placed at the centre.
Four particles of equal masses M move along a circle of radius R under the action of their mutual gravitational attraction. Find the speed of each particle.
Explain the following:
People often shake the branches of a tree for getting down its fruits.
How will the force of gravitation between two objects change if the distance between them is:
Infinite
How will the force of gravitation between two objects change if the distance between them is:
Almost zero
The force of attraction between any two material objects is called __________.
Where will you weigh more: at the moon's surface or at the earth's surface?
What is meant by the equation :
`g= Gxxm/r^2`
where the symbols have their usual meanings.
Answer the following question.
State Newton’s law of gravitation and express it in vector form.
The gravitational force between two bodies is directly proportional to the product of the masses of those bodies and is _______ of the distance between them.
For the weight of body of mass 5 kg to be zero on equator of the earth, angular velocity of the earth must be (The radius of earth = 6400 km, acceleration due to gravity = 10 m/s2).
The gravitational force between a hollow spherical shell (of radius R and uniform density) and a point mass is F. Show the nature of F vs r graph where r is the distance of the point from the centre of the hollow spherical shell of uniform density.
Six point masses of mass m each are at the vertices of a regular hexagon of side l. Calculate the force on any of the masses.
If three equal masses m are placed at the three vertices of an equilateral triangle of side 1/m then what force acts on a particle of mass 2m placed at the centroid?
