Advertisements
Advertisements
प्रश्न
A vertical pole of a length 6 m casts a shadow 4m long on the ground and at the same time a tower casts a shadow 28 m long. Find the height of the tower.
उत्तर १
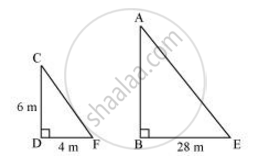
Let AB and CD be a tower and a pole respectively.
Let the shadow of BE and DF be the shadow of AB and CD respectively.
At the same time, the light rays from the sun will fall on the tower and the pole at the same angle.
Therefore, ∠DCF = ∠BAE
And, ∠DFC = ∠BEA
∠CDF = ∠ABE ...(Tower and pole are vertical to the ground)
∴ ΔABE ∼ ΔCDF ...(AAA similarity criterion)
⇒ `("AB")/("CB") = ("BE")/("DF")`
⇒ `("AB")/(6"m") = 28/4`
⇒ AB = 42 m
Therefore, the height of the tower will be 42 metres.
उत्तर २
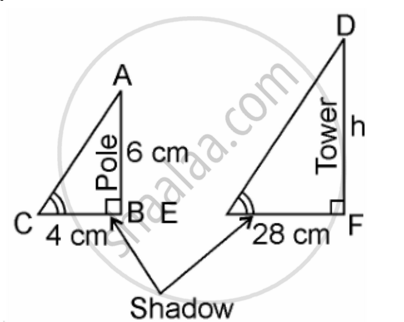
Length of the vertical pole = 6m ...(Given)
Shadow of the pole = 4 m ...(Given)
Let Height of tower = h m
Length of shadow of the tower = 28 m ...(Given)
In ΔABC and ΔDEF,
∠C = ∠E ...(angular elevation of sum)
∠B = ∠F = 90°
∴ ΔABC ~ ΔDEF ...(By AA similarity criterion)
∴ `"AB"/"DF" = "BC"/"EF"` ...(If two triangles are similar corresponding sides are proportional)
∴ `6/h = 4/28`
⇒ `h = (6×28)/4`
⇒ h = 6 × 7
⇒ h = 42 m
Hence, the height of the tower is 42 m.
APPEARS IN
संबंधित प्रश्न
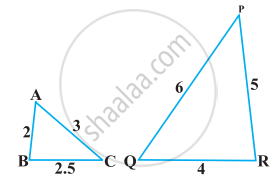
State which pair of triangles in the given figure are similar. Write the similarity criterion used by you for answering the question, and also write the pairs of similar triangles in the symbolic form:
S and T are point on sides PR and QR of ΔPQR such that ∠P = ∠RTS. Show that ΔRPQ ∼ ΔRTS.
In the following figure, if ΔABE ≅ ΔACD, show that ΔADE ∼ ΔABC.
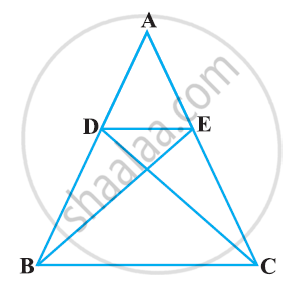
In the following figure, altitudes AD and CE of ΔABC intersect each other at the point P. Show that:
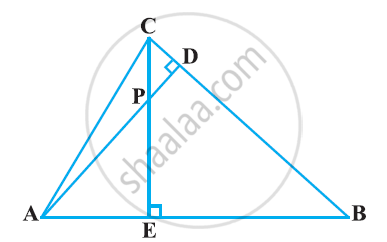
ΔAEP ∼ ΔADB
Sides AB and AC and median AD of a triangle ABC are respectively proportional to sides PQ and PR and median PM of another triangle PQR. Show that ΔABC ~ ΔPQR.
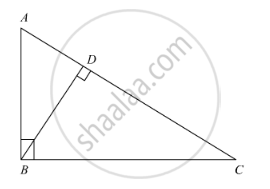
In the following Figure, ∠ABC = 90° and BD ⊥ AC. If AB = 5.7 cm, BD = 3.8 cm and CD = 5.4 cm, find BC.
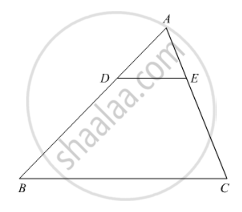
In the following Figure, DE || BC such that AE = (1/4) AC. If AB = 6 cm, find AD.
The sides of certain triangles are given below. Determine which of them right triangles are.
1.6cm, 3.8cm, 4cm
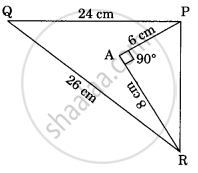
In the given figure, PQ = 24 cm, QR = 26 cm ∠PAR = 90°, PA = 6 cm, and AR = 8 cm, the degree measure of ∠QPR is ______.
It is given that ΔDEF ~ ΔRPQ. Is it true to say that ∠D = ∠R and ∠F = ∠P? Why?
In figure, if AB || DC and AC and PQ intersect each other at the point O, prove that OA . CQ = OC . AP
Areas of two similar triangles are 36 cm2 and 100 cm2. If the length of a side of the larger triangle is 20 cm, find the length of the corresponding side of the smaller triangle.
A 15 metres high tower casts a shadow 24 metres long at a certain time and at the same time, a telephone pole casts a shadow 16 metres long. Find the height of the telephone pole.
If in two triangles ABC and PQR, `(AB)/(QR) = (BC)/(PR) = (CA)/(PQ)`, then ______.
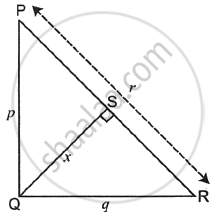
In the given figure, ΔPQR is a right-angled triangle with ∠PQR = 90°. QS is perpendicular to PR. Prove that pq = rx.
In the given figure, ΔLMN is similar to ΔPQR. To find the measure of ∠N, complete the following activity.

Given: ΔLMN ∼ ΔPQR
Since ΔLMN ∼ ΔPQR, therefore, corresponding angles are equal.
So, ∠L ≅ `square`
⇒ ∠L = `square`
We know, the sum of angles of a triangle = `square`
∴ ∠L + ∠M + ∠N = `square`
Substituting the values of ∠L and ∠M in equation (i),
`square` + `square` + ∠N = `square`
∠N + `square` = `square`
∠N = `square` – `square`
∠N = `square`
Hence, the measure of ∠N is `square`.
A tangent ADB is drawn to a circle at D whose centre is C. Also, PQ is a chord parallel to AB and ∠QDB = 50°. Find the value of ∠PDQ.

In the given figure, ∠ADC = ∠BCA; prove that ΔACB ∼ ΔADC. Hence find BD if AC = 8 cm and AD = 3 cm.
`square`ABCD is trapezium, AB || CD diagonals of trapezium intersects in point P.
Write the answers of the following questions:
- Draw the figure using the given information.
- Write any one pair of alternate angles and opposite angles.
- Write the names of similar triangles with the test of similarity.
