Advertisements
Advertisements
प्रश्न
उत्तर
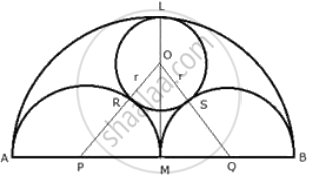
Let O, P and Q be the centers of the circle and semicircle.
Join OP and OQ.
OR = OS = r
And `AP = PM = MQ = QB = (AB)/4`
Now, `OP = OR + RP = r + (AB)/4` ...(Since PM = RP = radii of same circle)
Similarly, `OQ = OS + SQ = r + (AB)/4`
`OM = LM - OL = (AB)/2 - r`
Now in right ΔOPM,
OP2 = PM2 + OM2
`=> (r + (AB)/4)^2 = ((AB)/4)^2 + ((AB)/2 - r)^2`
`=> r^2 + (AB^2)/16 + (rAB)/2 = (AB^2)/16 + (AB^2)/4 + r^2 - rAB`
`=> (rAB)/2 = (AB^2)/4 - rAB`
`=> (AB^2)/4 = (rAB)/2 + rAB`
`=> (AB^2)/4 = (3rAB)/2`
`=> (AB)/4 = 3/2 r`
`=> AB = 3/2 rxx 4 = 6r`
Hence AB = 6 × r
APPEARS IN
संबंधित प्रश्न
Calculate the area of the shaded region, if the diameter of the semicircle is equal to 14 cm. Take `pi = 22/7`

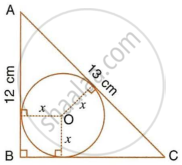
ABC is a right angles triangle with AB = 12 cm and AC = 13 cm. A circle, with centre O, has been inscribed inside the triangle.
Calculate the value of x, the radius of the inscribed circle.
Prove that the parallelogram, inscribed in a circle, is a rectangle.
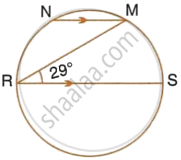
In the given figure, RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°. Calculate : ∠RNM
Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.
Prove that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.
In the figure, given below, AB and CD are two parallel chords and O is the centre. If the radius of the circle is 15 cm, fins the distance MN between the two chords of lengths 24 cm and 18 cm respectively.

Using ruler and a compass only construct a semi-circle with diameter BC = 7cm. Locate a point A on the circumference of the semicircle such that A is equidistant from B and C. Complete the cyclic quadrilateral ABCD, such that D is equidistant from AB and BC. Measure ∠ADC and write it down.
In the following figure, AD is the diameter of the circle with centre O. chords AB, BC and CD are equal. If ∠DEF = 110°, Calculate: ∠FAB.

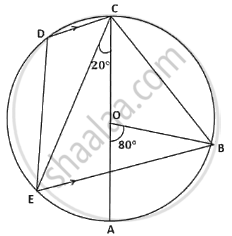
In the given figure AC is the diameter of the circle with centre O. CD is parallel to BE.
∠AOB = 80° and ∠ACE = 20°.
Calculate
- ∠BEC
- ∠BCD
- ∠CED