Advertisements
Advertisements
प्रश्न
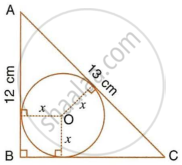
ABC is a right angles triangle with AB = 12 cm and AC = 13 cm. A circle, with centre O, has been inscribed inside the triangle.
Calculate the value of x, the radius of the inscribed circle.
उत्तर
In ΔABC, ∠B = 90°
OL ⊥ AB, OM ⊥ BC and ON ⊥ AC
LBNO is a square
LB = BN = OL = OM = ON = x
∴ AL = 12 – x
∴ AL = AN = 12 – x
Since ABC is a right triangle
AC2 = AB2 + BC2
`=>` 132 = 122 + BC2
`=>` 169 = 144 + BC2
`=>` BC2 = 25
`=>` BC = 5
∴ MC = 5 – x
But CM = CN
∴ CN = 5 – x
Now, AC = AN + NC
13 = (12 – x) + (5 – x)
13 = 17 – 2x
2x = 4
x = 2 cm
APPEARS IN
संबंधित प्रश्न
Prove that the rhombus, inscribed in a circle, is a square.
ABCD is a cyclic quadrilateral in which AB is parallel to DC and AB is a diameter of the circle. Given ∠BED = 65°; calculate :
- ∠DAB,
- ∠BDC.

In the given figure, PQ is a diameter. Chord SR is parallel to PQ. Given that ∠PQR = 58°,
Calculate:
- ∠RPQ,
- ∠STP.

In the figure, given below, AB and CD are two parallel chords and O is the centre. If the radius of the circle is 15 cm, fins the distance MN between the two chords of lengths 24 cm and 18 cm respectively.

Using ruler and a compass only construct a semi-circle with diameter BC = 7cm. Locate a point A on the circumference of the semicircle such that A is equidistant from B and C. Complete the cyclic quadrilateral ABCD, such that D is equidistant from AB and BC. Measure ∠ADC and write it down.
In the given figure, AB is a diameter of the circle. Chord ED is parallel to AB and ∠EAB = 63°. Calculate : ∠BCD.
In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate : ∠DBA
Also, show that the ΔAOD is an equilateral triangle.
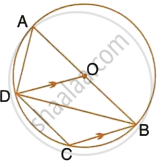
In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate : ∠DBC
Also, show that the ΔAOD is an equilateral triangle.

In the given figure, AB is a diameter of the circle with centre O. DO is parallel to CB and ∠DCB = 120°.
Calculate : ∠ADC
Also, show that the ΔAOD is an equilateral triangle.
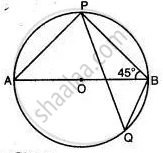
In the given figure, O is the centre of the circle and ∠PBA = 45°. Calculate the value of ∠PQB.