Advertisements
Advertisements
प्रश्न
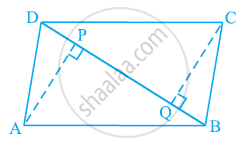
ABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD (See the given figure). Show that
- ΔAPB ≅ ΔCQD
- AP = CQ
उत्तर
i. In ΔAPB and ΔCQD,
∠APB = ∠CQD ...(Each 90°)
AB = CD ...(Opposite sides of parallelogram ABCD)
∠ABP = ∠CDQ ...(Alternate interior angles for AB || CD)
∴ ΔAPB ≅ ΔCQD ...(By AAS congruency)
ii. By using the above result
ΔAPB ≅ ΔCQD, we obtain
AP = CQ ...(By CPCT)
APPEARS IN
संबंधित प्रश्न
In the following figures, ABCD is a parallelogram.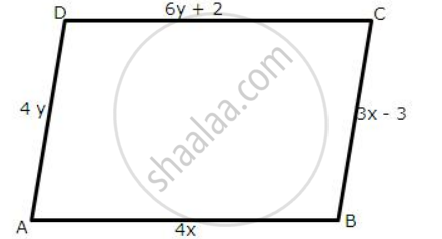
find the values of x and y.
In the figure, ABCD is a parallelogram and APD is an equilateral triangle of side 80cm, Calculate the area of parallelogram ABCD.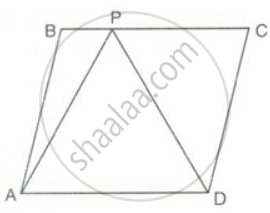
In the figure, AE = BE. Prove that the area of triangle ACE is equal in area to the parallelogram ABCD.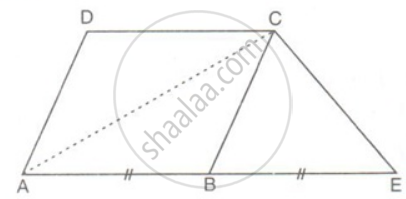
AD is a median of a ΔABC.P is any point on AD. Show that the area of ΔABP is equal to the area of ΔACP.
Find the area of quadrilateral, whose diagonals of lengths 18 cm and 13 cm intersect each other at right angle.
A rectangular hall of 40m by 24m is covered with carpets of size 6m x 4m. Find the number of carpets required to cover the hall.
A rectangular field is 80m long and 50m wide. A 4m wide runs through the centre of the field parallel to the length and breadth of the field. Find the total area of the roads.
The diagonals of a square are perpendicular to one another.
All the sides of a parallelogram are of equal length.
Name polygon.

Make two more examples of this.
