Advertisements
Advertisements
प्रश्न
ABCD is a trapezium in which AB || DC and P and Q are points on AD and BC, respectively such that PQ || DC. If PD = 18 cm, BQ = 35 cm and QC = 15 cm, find AD.
उत्तर
Given, a trapezium ABCD in which AB || DC.
P and Q are points on AD and BC, respectively such that PQ || DC.
Thus, AB || PQ || DC.
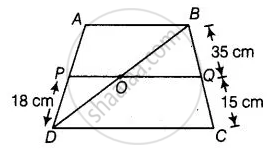
Join BD.
In ΔABD,
PO || AB ...[∵ PQ || AB]
By basic proportionality theorem,
`("DP")/("AP") = ("DO")/("OB")` ...(i)
In ΔBDC,
OQ || DC ...[∵ PQ || DC]
By basic proportionality theorem,
`("BQ")/("QC") = ("OB")/("OD")`
⇒ `("QC")/("BQ") = ("OD")/("OB")` ...(ii)
From equation (i) and (ii),
`("DP")/("AP") = ("QC")/("BQ")`
⇒ `18/("AP") = 15/35`
⇒ AP = `(18 xx 35)/15` = 42
∴ AD = AP + DP
= 42 + 18
= 60 cm
APPEARS IN
संबंधित प्रश्न
In ΔABC, D and E are points on the sides AB and AC respectively such that DE || BC
If AD = 2.5 cm, BD = 3.0 cm and AE = 3.75 cm, find the length of AC.
In a ΔABC, D and E are points on the sides AB and AC respectively. For the following case show that DE || BC
AB = 10.8 cm, BD = 4.5 cm, AC = 4.8 cm and AE = 2.8 cm.
M and N are points on the sides PQ and PR respectively of a ΔPQR. For the following case, state whether MN || QR
PM = 4cm, QM = 4.5 cm, PN = 4 cm and NR = 4.5 cm
D and E are points on the sides AB and AC respectively of a ΔABC such that DE║BC.
If `(AD)/(DB) = 4/7` and AC = 6.6cm, find AE.
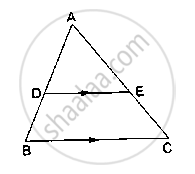
ΔABC and ΔDBC lie on the same side of BC, as shown in the figure. From a point P on BC, PQ||AB and PR||BD are drawn, meeting AC at Q and CD at R respectively. Prove that QR||AD.
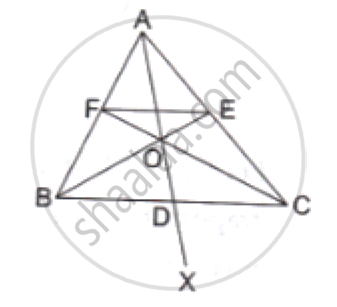
State and converse of Thale’s theorem.
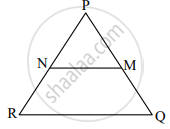
In ΔPQR, NM || RQ. If PM = 15, MQ = 10, NR = 8, then find PN.
From fig., seg PQ || side BC, AP = x + 3, PB = x – 3, AQ = x + 5, QC = x – 2, then complete the activity to find the value of x.
In ΔPQB, PQ || side BC
`"AP"/"PB" = "AQ"/(["______"])` ...[______]
`(x + 3)/(x - 3) = (x + 5)/(["______"])`
(x + 3) [______] = (x + 5)(x – 3)
x2 + x – [______] = x2 + 2x – 15
x = [______]
In fig., PS = 2, SQ = 6, QR = 5, PT = x and TR = y. Then find the pair of value of x and y such that ST || side QR.
Prove that If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio. In the figure, find EC if `(AD)/(DB) = (AE)/(EC)` using the above theorem.

