Question

AD is an altitude of an equilateral triangle ABC. On AD as base, another equilateral triangle ADE is constructed. Prove that Area (ΔADE): Area (ΔABC) = 3: 4

Answer 1

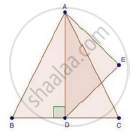
We have,

ΔABC is an equilateral triangle

Then, AB = BC = AC

Let, AB = BC = AC = 2x

Since, AD ⊥ BC then BD = DC = x

In ΔADB, by Pythagoras theorem

𝐴𝐵² = (2𝑥)² − (𝑥)²

⇒ 𝐴𝐷² = 4𝑥² − 𝑥² = 3𝑥²

⇒ 𝐴𝐷 = `sqrt3`𝑥 cm

Since, ΔABC and ΔADE both are equilateral triangles then they are equiangular

∴ ΔABC ~ ΔADE [By AA similarity]

By area of similar triangle theorem

`("area"(triangleADE))/("area"(triangleABC))="AD"^2/"AB"^2`

`=(sqrt3x)^2/(2x)^2`

`=(3x^2)/(4x^2)`

`=3/4`