Advertisements
Advertisements
प्रश्न
If the perimeter of two similar triangles is in the ratio 2 : 3, what is the ratio of their sides?
पर्याय
4 : 9
2 : 3
`sqrt(2)` : `sqrt(3)`
3 : 2
उत्तर
2 : 3
Explanation:
Let two similar triangles be ABC and DEF.
Since, the triangles are similar,
∴ `("Perimeter" (ΔABC))/("Perimeter" (ΔDEF)) = (AB)/(DE)`
According to the question,
`("Perimeter" (ΔABC))/("Perimeter" (ΔDEF)) = 2/3`
∴ `(AB)/(DE) = 2/3`
Thus, the ratio of sides is 2 : 3.
APPEARS IN
संबंधित प्रश्न
The areas of two similar triangles ∆ABC and ∆PQR are 25 cm2 and 49 cm2 respectively. If QR = 9.8 cm, find BC
If ∆ABC is similar to ∆DEF such that ∆DEF = 64 cm2 , DE = 5.1 cm and area of ∆ABC = 9 cm2 . Determine the area of AB
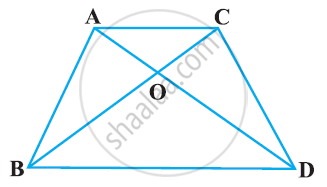
In a trapezium ABCD, O is the point of intersection of AC and BD, AB || CD and AB = 2 × CD. If the area of ∆AOB = 84 cm2 . Find the area of ∆COD
Prove that the area of the triangle BCE described on one side BC of a square ABCD as base is one half the area of the similar Triangle ACF described on the diagonal AC as base
In Figure, ABC and DBC are two triangles on the same base BC. If AD intersects BC at O, show that `(ar(ABC))/(ar(DBC)) = (AO)/(DO)`
If the areas of two similar triangles are equal, prove that they are congruent.
The areas of two similar triangles are 169 cm2 and 121 cm2 respectively. If the longest side of the larger triangle is 26 cm, find the longest side of the smaller triangle.
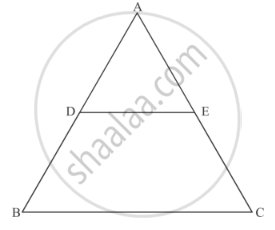
In Figure, DE || BC If DE = 4cm, BC = 8 cm and Area (ΔADE) = 25 cm2, find the area of ΔABC.
The areas of two similar triangles are 100 cm2 and 49 cm2 respectively. If the altitude the bigger triangle is 5 cm, find the corresponding altitude of the other.
In ΔABC, PQ is a line segment intersecting AB at P and AC at Q such that PQ || BC and PQ divides ΔABC into two parts equal in area. Find `(BP)/(AB)`
AD is an altitude of an equilateral triangle ABC. On AD as base, another equilateral triangle ADE is constructed. Prove that Area (ΔADE): Area (ΔABC) = 3: 4
If ∆ABC ~ ∆PQR, A (∆ABC) = 80, A (∆PQR) = 125, then fill in the blanks. \[\frac{A\left( ∆ ABC \right)}{A\left( ∆ . . . . \right)} = \frac{80}{125} \therefore \frac{AB}{PQ} = \frac{......}{......}\]
Prove that in a right-angle triangle, the square of the hypotenuse is equal to the sum of squares of the other two sides.
The perpendicular from A on side BC of a Δ ABC meets BC at D such that DB = 3CD. Prove that 2AB2 = 2AC2 + BC2.
The ratio of corresponding sides of similar triangles is 3 : 5, then find the ratio of their areas.
If ∆XYZ ~ ∆PQR and A(∆XYZ) = 25 cm2, A(∆PQR) = 4 cm2 then XY : PQ = ?
ΔABC ~ ΔPQR. In ΔABC, AB = 5.4 cm, BC = 4.2 cm, AC = 6.0 cm, AB:PQ = 3:2, then construct ΔABC and ΔPQR.
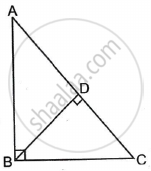
In the adjoining figure, ΔADB ∼ ΔBDC. Prove that BD2 = AD × DC.