Advertisements
Advertisements
प्रश्न
An ideal gas is pumped into a rigid container having diathermic walls so that the temperature remains constant. In a certain time interval, the pressure in the container is doubled. Is the internal energy of the contents of the container also doubled in the interval ?
उत्तर
An ideal gas is continuously being pumped into the container. Therefore, the number of moles, n are continuously increasing. In a certain interval,
Pressure, P2 = 2P1
n2 = 2n1
Thus, internal energy, U = nCvT will double as the number of moles get doubled.
APPEARS IN
संबंधित प्रश्न
A cylinder containing a gas is lifted from the first floor to the second floor. What is the amount of work done on the gas? What is the amount of work done by the gas? Is the internal energy of the gas increased? Is the temperature of the gas increased?
A force F is applied on a block of mass M. The block is displaced through a distance d in the direction of the force. What is the work done by the force on the block? Does the internal energy change because of this work?
Can work be done by a system without changing its volume?
Consider the following two statements.
(A) If heat is added to a system, its temperature must increase.
(B) If positive work is done by a system in a thermodynamic process, its volume must increase.
The pressure p and volume V of an ideal gas both increase in a process.
(a) Such a process is not possible.
(b) The work done by the system is positive.
(c) The temperature of the system must increase.
(d) Heat supplied to the gas is equal to the change in internal energy.
A 100 kg lock is started with a speed of 2.0 m s−1 on a long, rough belt kept fixed in a horizontal position. The coefficient of kinetic friction between the block and the belt is 0.20. (a) Calculate the change in the internal energy of the block-belt system as the block comes to a stop on the belt. (b) Consider the situation from a frame of reference moving at 2.0 m s−1 along the initial velocity of the block. As seen from this frame, the block is gently put on a moving belt and in due time the block starts moving with the belt at 2.0 m s−1. calculate the increase in the kinetic energy of the block as it stops slipping past the belt. (c) Find the work done in this frame by the external force holding the belt.
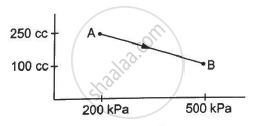
A gas is taken along the path AB as shown in figure. If 70 cal of heat is extracted from the gas in the process, calculate the change in the internal energy of the system.
A mixture of fuel and oxygen is burned in a constant-volume chamber surrounded by a water bath. It was noticed that the temperature of water is increased during the process. Treating the mixture of fuel and oxygen as the system,
- Has heat been transferred?
- Has work been done?
- What is the sign of ∆U?
A system releases 130 kJ of heat while 109 kJ of work is done on the system. Calculate the change in internal energy.
Which of the following is correct, when the energy is transferred to a system from its environment?
Which of the following system freely allows the exchange of energy and matter with its environment?
Two samples A and B, of a gas at the same initial temperature and pressure are compressed from volume V to V/2; A isothermally and B adiabatically. The final pressure of A will be ______.
In insulated systems, the amount of external work done by the gas is proportional to:
n mole of a perfect gas undergoes a cyclic process ABCA (see figure) consisting of the following processes:
A `→` B: Isothermal expansion at temperature T so that the volume is doubled from V1 to V2 = 2V1 and pressure changes from P1 to P2.
B `→` C: Isobaric compression at pressure P2 to initial volume V1.
C `→` A: Isochoric change leading to change of pressure from P2 to P1.
Total workdone in the complete cycle ABCA is ______.

A gas is compressed at a constant pressure of 50 N/m2 from a volume of 10 m3 to a volume of 4 m3. Energy of 100 J is then added to the gas by heating. Its internal energy is ______.
The internal energy of one mole of argon is ______.
The molar specific heat of He at constant volume is 12.47 J/mol.K. Two moles of He are heated at constant pressure. So the rise in temperature is 10 K. Find the increase in internal energy of the gas.
A system releases 125 kJ of heat while 104 kJ work is done on the system. Calculate the change in internal energy.
