Advertisements
Advertisements
प्रश्न
Arrange the sides of the following triangles in an ascending order:
ΔABC, ∠A = 45°, ∠B = 65°.
उत्तर
In ΔABC,
∠A + ∠B + ∠C = 180°
45° + 65° + ∠C = 180°
110° +∠C = 180°
∠C = 180° - 110°
∠C = 70°
Hence, ∠A = 45°, ∠B = 65°, ∠C = 70°
45° < 65° < 70°
Hence, ascending order of the angles in the
given triangle is ∠A < ∠B < ∠C.
Hence, ascending order of sides in triangle
BC, AC, AB.
APPEARS IN
संबंधित प्रश्न
From the following figure, prove that: AB > CD.
In the following figure, ∠BAC = 60o and ∠ABC = 65o.

Prove that:
(i) CF > AF
(ii) DC > DF
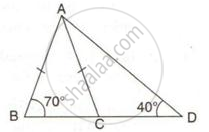
In the following figure, write BC, AC, and CD in ascending order of their lengths.
"Caste inequalities are still prevalent in India." Examine the statement.
Name the smallest angle in each of these triangles:
In ΔABC, AB = 6.2cm, BC = 5.6cm and AC = 4.2cm
In a triangle ABC, BC = AC and ∠ A = 35°. Which is the smallest side of the triangle?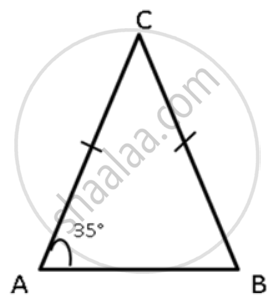
ΔABC is isosceles with AB = AC. If BC is extended to D, then prove that AD > AB.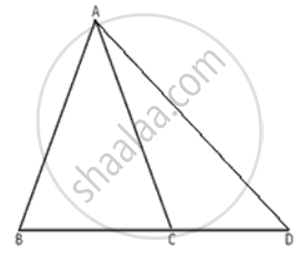
Prove that the perimeter of a triangle is greater than the sum of its three medians.
In ABC, P, Q and R are points on AB, BC and AC respectively. Prove that AB + BC + AC > PQ + QR + PR.
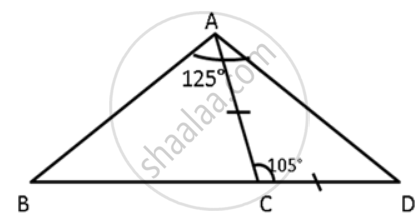
In ΔABC, BC produced to D, such that, AC = CD; ∠BAD = 125° and ∠ACD = 105°. Show that BC > CD.