Advertisements
Advertisements
प्रश्न
ΔABC is isosceles with AB = AC. If BC is extended to D, then prove that AD > AB.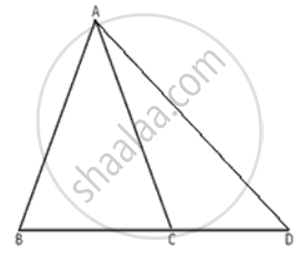
उत्तर
Using the exterior angle and property in ΔACD, we have
∠ACB = ∠CDA + ∠CAD
⇒ ∠ACB > ∠CDA ------(1)
Now, AB = AC
Now, ∠ACB = ∠ABC ------(2)
From (1) and (2)
∠ABC > ∠CDA
It is known that, in a triangle, the greater angle has the longer side opposite to it.
Now, In ΔABD, er have ∠ABC > ∠CDA
∴ AD > AB.
APPEARS IN
संबंधित प्रश्न
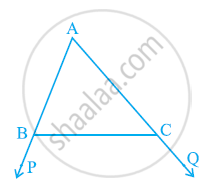
In the given figure sides AB and AC of ΔABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
In a huge park people are concentrated at three points (see the given figure):

A: where there are different slides and swings for children,
B: near which a man-made lake is situated,
C: which is near to a large parking and exit.
Where should an ice-cream parlour be set up so that maximum number of persons can approach it?
(Hint: The parlor should be equidistant from A, B and C)
In the following figure, write BC, AC, and CD in ascending order of their lengths.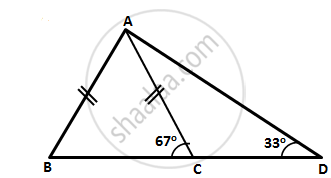
D is a point in side BC of triangle ABC. If AD > AC, show that AB > AC.
Name the greatest and the smallest sides in the following triangles:
ΔABC, ∠ = 56°, ∠B = 64° and ∠C = 60°.
For any quadrilateral, prove that its perimeter is greater than the sum of its diagonals.
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that : PN < RN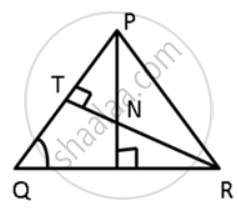
In ΔPQR, PS ⊥ QR ; prove that: PQ > QS and PQ > PS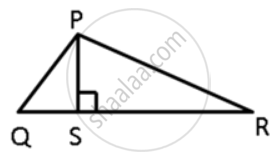
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that RT < QT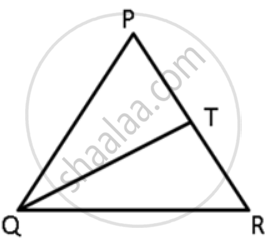
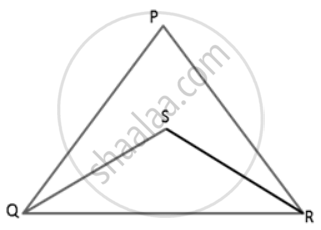
In ΔPQR is a triangle and S is any point in its interior. Prove that SQ + SR < PQ + PR.