Advertisements
Advertisements
प्रश्न
In ΔABC, the exterior ∠PBC > exterior ∠QCB. Prove that AB > AC.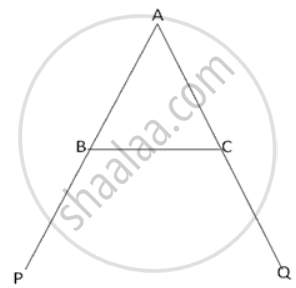
उत्तर
It is given that ∠PBC > ∠QCB -------(1)
∠PBC + ∠ABC = 180 ...[Linear pair angles]
⇒ ∠PBC = 180 - ∠ABC
Similarly, ∠QCB = 180 - ∠ACB
From (1) and (2)
180 - ∠ABC > 180 - ∠ACB
⇒ -∠ABC > - ∠ACB
⇒ ABC < ∠ACB or ∠ACB > ∠ABC
It is known that in a triangle, the greater angle has the longer side opposite to it.
∴ AB > AC.
APPEARS IN
संबंधित प्रश्न
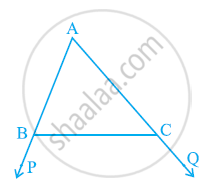
In the given figure sides AB and AC of ΔABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
How had the position of women improved in our country since independence ? Explain with examples.
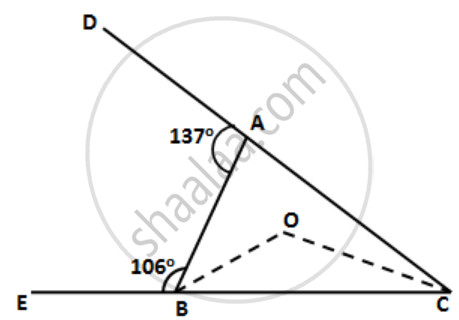
Arrange the sides of ∆BOC in descending order of their lengths. BO and CO are bisectors of angles ABC and ACB respectively.
Name the greatest and the smallest sides in the following triangles:
ΔABC, ∠ = 56°, ∠B = 64° and ∠C = 60°.
Arrange the sides of the following triangles in an ascending order:
ΔABC, ∠A = 45°, ∠B = 65°.
Name the smallest angle in each of these triangles:
In ΔABC, AB = 6.2cm, BC = 5.6cm and AC = 4.2cm
Name the smallest angle in each of these triangles:
In ΔXYZ, XY = 6.2cm, XY = 6.8cm and YZ = 5cm
ABCD is a trapezium. Prove that: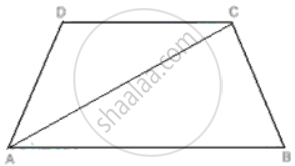
CD + DA + AB > BC.
In ΔPQR, PS ⊥ QR ; prove that: PQ > QS and PR > PS
Prove that in an isosceles triangle any of its equal sides is greater than the straight line joining the vertex to any point on the base of the triangle.
