Advertisements
Advertisements
प्रश्न
In the given figure, T is a point on the side PR of an equilateral triangle PQR. Show that RT < QT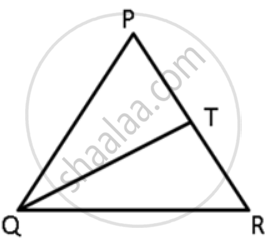
उत्तर
In ΔPQR,
PQ = QR = PR
⇒ ∠P = Q = ∠ = 60°
In ΔTQR,
∠TQR < 60°
∴ ∠TQR < ∠R
∴ RT < QT.
APPEARS IN
संबंधित प्रश्न
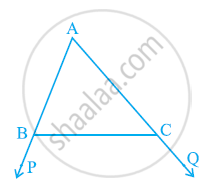
In the given figure sides AB and AC of ΔABC are extended to points P and Q respectively. Also, ∠PBC < ∠QCB. Show that AC > AB.
AB and CD are respectively the smallest and longest sides of a quadrilateral ABCD (see the given figure). Show that ∠A > ∠C and ∠B > ∠D.

ABC is a triangle. Locate a point in the interior of ΔABC which is equidistant from all the vertices of ΔABC.
How had the position of women improved in our country since independence ? Explain with examples.
If two sides of a triangle are 8 cm and 13 cm, then the length of the third side is between a cm and b cm. Find the values of a and b such that a is less than b.
In the following figure ; AC = CD; ∠BAD = 110o and ∠ACB = 74o.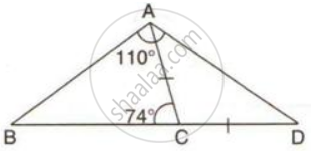
Prove that: BC > CD.
Name the greatest and the smallest sides in the following triangles:
ΔXYZ, ∠X = 76°, ∠Y = 84°.
Arrange the sides of the following triangles in an ascending order:
ΔDEF, ∠D = 38°, ∠E = 58°.
ΔABC is isosceles with AB = AC. If BC is extended to D, then prove that AD > AB.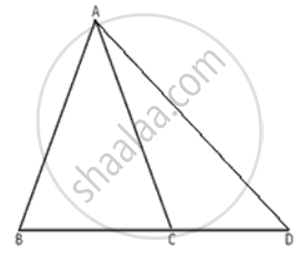
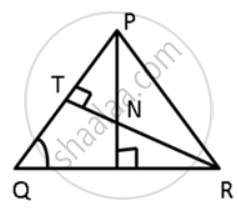
In the given figure, ∠QPR = 50° and ∠PQR = 60°. Show that : PN < RN