Advertisements
Advertisements
प्रश्न
Describe the locus of a point in rhombus ABCD, so that it is equidistant from
- AB and BC;
- B and D.
उत्तर
i.

The locus of the point in a rhombus ABCD which is equidistant from AB and BC will be the diagonal BD.
ii.
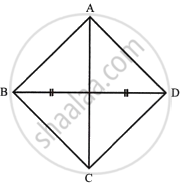
The locus of the point in a rhombus ABCD which is equidistant from B and D will be the diagonal AC.
APPEARS IN
संबंधित प्रश्न
Draw an angle ABC = 75°. Draw the locus of all the points equidistant from AB and BC.
Describe the locus of the moving end of the minute hand of a clock.
Describe the locus of the centres of all circles passing through two fixed points.
Sketch and describe the locus of the vertices of all triangles with a given base and a given altitude.
A straight line AB is 8 cm long. Draw and describe the locus of a point which is:
- always 4 cm from the line AB.
- equidistant from A and B.
Mark the two points X and Y, which are 4 cm from AB and equidistant from A and B. Describe the figure AXBY.
In Δ ABC, the perpendicular bisector of AB and AC meet at 0. Prove that O is equidistant from the three vertices. Also, prove that if M is the mid-point of BC then OM meets BC at right angles.
In a quadrilateral PQRS, if the bisectors of ∠ SPQ and ∠ PQR meet at O, prove that O is equidistant from PS and QR.
Show that the locus of the centres of all circles passing through two given points A and B, is the perpendicular bisector of the line segment AB.
ΔPBC, ΔQBC and ΔRBC are three isosceles triangles on the same base BC. Show that P, Q and R are collinear.
Use ruler and compasses for the following question taking a scale of 10 m = 1 cm. A park in a city is bounded by straight fences AB, BC, CD and DA. Given that AB = 50 m, BC = 63 m, ∠ABC = 75°. D is a point equidistant from the fences AB and BC. If ∠BAD = 90°, construct the outline of the park ABCD. Also locate a point P on the line BD for the flag post which is equidistant from the corners of the park A and B.
