Advertisements
Advertisements
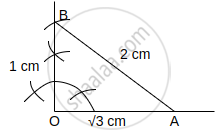
प्रश्न
Draw a line segment of length `sqrt3` cm.
उत्तर
Construct a right angled triangle OAB, in which

∠A = 90°, OB = 2 cm and AB = 1 cm
Using OA2 + AB2 = OB2
we get: OA = `sqrt3` cm
APPEARS IN
संबंधित प्रश्न
Rationalize the denominator.
`1/(sqrt 3 - sqrt 2)`
Simplify : `sqrt18/[ 5sqrt18 + 3sqrt72 - 2sqrt162]`
Simplify the following
`(3)/(5 - sqrt(3)) + (2)/(5 + sqrt(3)`
Simplify the following
`(4 + sqrt(5))/(4 - sqrt(5)) + (4 - sqrt(5))/(4 + sqrt(5)`
Simplify the following :
`(3sqrt(2))/(sqrt(6) - sqrt(3)) - (4sqrt(3))/(sqrt(6) - sqrt(2)) + (2sqrt(3))/(sqrt(6) + 2)`
In the following, find the values of a and b:
`(sqrt(2) + sqrt(3))/(3sqrt(2) - 2sqrt(3)) = "a" - "b"sqrt(6)`
If x = `((sqrt(3) + 1))/((sqrt(3) - 1)` and y = `((sqrt(3) - 1))/((sqrt(3) + 1)`, find the values of
x2 + y2
Simplify:
`(sqrt(x^2 + y^2) - y)/(x - sqrt(x^2 - y^2)) ÷ (sqrt(x^2 - y^2) + x)/(sqrt(x^2 + y^2) + y)`
Show that Negative of an irrational number is irrational.
Show that: `(4 - sqrt5)/(4 + sqrt5) + 2/(5 + sqrt3) + (4 + sqrt5)/(4 - sqrt5) + 2/(5 - sqrt3) = 52/11`
