Advertisements
Advertisements
प्रश्न
Find the length of a chord which is at a distance of 5 cm from the centre of a circle ofradius 10 cm.
उत्तर
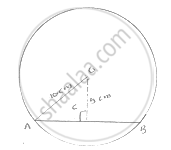
Given that
Distance (OC)=5cm
Radius of circle (OA)10cm
In OCA by Pythagoras theorem
WRK, the perpendicular from center to chord bisects the chord
Then chord AB=8.66+8.66
= 17.32 cm
APPEARS IN
संबंधित प्रश्न
Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord.
If two equal chords of a circle intersect within the circle, prove that the segments of one chord are equal to corresponding segments of the other chord.
If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
If a line intersects two concentric circles (circles with the same centre) with centre O at A, B, C, and D, prove that AB = CD (see given figure).

Three girls Reshma, Salma and Mandip are playing a game by standing on a circle of radius 5m drawn in a park. Reshma throws a ball to Salma, Salma to Mandip, Mandip to Reshma. If the distance between Reshma and Salma and between Salma and Mandip is 6m each, what is the distance between Reshma and Mandip?
true or false
If a circle is divided into three equal arcs each is a major arc.
Two chords AB and CD of lengths 5 cm and 11 cm respectively of a circle are parallel to each other and are opposite side of its center. If the distance between AB and CD is 6 cm. Find the radius of the circle.
In a circle with centre O, AB and CD are two diameters perpendicular to each other. The length of chord AC is
Two congruent circles with centres O and O′ intersect at two points A and B. Then ∠AOB = ∠AO′B.
AB and AC are two chords of a circle of radius r such that AB = 2AC. If p and q are the distances of AB and AC from the centre, prove that 4q2 = p2 + 3r2.
