Advertisements
Advertisements
प्रश्न
Find the ratio in which the point R ( 1, 5) divides the line segment joining the points S (-2, -1) and T (5, 13).
उत्तर
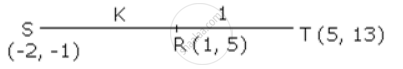
Let R divides the line segment ST in the ratio k : 1. Coordinates of R
R ( x, y) = R ( 1, 5)
R `((5"k" - 2)/("k" + 1) , (13 "k" - 1)/("k" + 1))` = R (1 , 5)
`(5"k" - 2)/("k" +1) = 1`
5 k - 2 = k + 1
4 k = 3
k = `3/4`
Hence, required ratiois k :1 = 3 : 4.
APPEARS IN
संबंधित प्रश्न
Find the coordinates of points which trisect the line segment joining (1, –2) and (–3, 4)
If the point C (–1, 2) divides internally the line segment joining A (2, 5) and B in ratio 3 : 4, find the coordinates of B
If the coordinates of the mid-points of the sides of a triangle are (1, 2) (0, –1) and (2, 1). Find the coordinates of its vertices.
Find the lengths of the medians of a ∆ABC whose vertices are A(7, –3), B(5,3) and C(3,–1)
Three vertices of a parallelogram are (a+b, a-b), (2a+b, 2a-b), (a-b, a+b). Find the fourth vertex.
In what ratio is the join of (4, 3) and (2, –6) divided by the x-axis? Also, find the co-ordinates of the point of intersection.
Q is a point on the line segment AB. The coordinates of A and B are (2, 7) and (7, 12) along the line AB so that AQ = 4BQ. Find the coordinates of Q.
Show that the line segment joining the points (-3, 10) and (6, -5) is trisected by the coordinates axis.
Find the ratio in which Y-axis divides the point A(3, 5) and point B(– 6, 7). Find the coordinates of the point
If the points A(1, –2), B(2, 3) C(a, 2) and D(– 4, –3) form a parallelogram, find the value of a and height of the parallelogram taking AB as base.
