Advertisements
Advertisements
प्रश्न
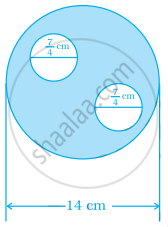
Find the area of the shaded region:
उत्तर
∵ Diameter of complete circle = 14 cm
∴ Radius = `14/2` = 7 cm .....`[∵ "Radius" = "Diameter"/2]`
So, area of complete circle = πr2 = `22/7 xx 7 xx 7` = 154 cm2
∵ Diameter of small circle = `7/4`cm
∴ Radius = `7/(4 xx 2) = 7/8`cm
∴ Area of two small circles = 2 × πr2
= `2 xx 22/7 xx 7/8 xx 7/8`
= `77/16`cm2
∴ Area of shaded region = Area of complete circle – Area of two small circles
= `154 - 77/16`
= `(154 xx 16 - 77)/16` ......[Taking LCM]
= `(2464 - 77)/16`
= `2387/16`
= `149 3/16` cm2
Hence, the area of shaded region is `149 3/16`cm2.
APPEARS IN
संबंधित प्रश्न
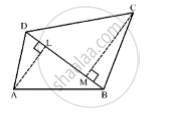
In the given figure ABCD is quadrilateral in which diagonal BD = 24 cm, AL ⊥ BD and CM ⊥ BD such that AL = 9cm and CM = 12 cm. Calculate the area of the quadrilateral.
In the following figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the (i) quadrant OACB (ii) shaded region.

In the following figure, ABC is an equilateral triangle of side 8 cm. A, B and C are the centres of circular arcs of radius 4 cm. Find the area of the shaded region correct upto 2 decimal places. (Take π =3.142 and`sqrt3` = 1.732).

If the sum of the areas of two circles with radii r1 and r2 is equal to the area of a circle of radius r, then \[r_1^2 + r_2^2\]
In the following figure, the area of the segment PAQ is

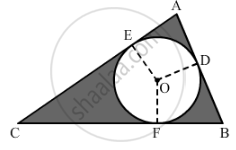
In the given figure, ∆ABC is right-angled at A. Find the area of the shaded region if AB = 6 cm, BC = 10 cm and O is the centre of the incircle of ∆ABC.
The diameter of a wheel is 40 cm. How many revolutions will it make in covering 176 m?
A square is inscribed in a circle of radius 7 cm. Find the area of the square.
The area of a circle is 1386 sq.cm; find its circumference.
Find the area of the dining table whose diameter is 105 cm
