Advertisements
Advertisements
प्रश्न
Find the equation of the straight line perpendicular to 5x – 2y = 8 and which passes through the mid-point of the line segment joining (2, 3) and (4, 5).
उत्तर
5x - 2y = 8
2y = 5x - 8
⇒ y = `(5)/(2) x - 4`
y = mx + c
∴ m1 = `(5)/(2)`
Since lines are perpendicular to each other
∴ m1 x m2 = -1
`(5)/(2) xx m_2` = -1
m2 = `-1 + (2)/(5)`
m2 = `-(2)/(5)`
Coordinates of midpoints
= `(2 + 4)/(2),(3 + 5)/(2)`
Passing Point = (3, 4)
∴ Equation of line,
y - y1 = m(x - x1)
⇒ y - 4 = `(-2)/(5)(x - 3)`
⇒ 5y - 20 = -2x + 6
⇒ 2x + 5y = 26.
APPEARS IN
संबंधित प्रश्न
Find, which of the following points lie on the line x – 2y + 5 = 0 :
(–5, 0)
The line given by the equation `2x - y/3 = 7` passes through the point (k, 6); calculate the value of k.
The line segment joining the points (5, −4) and (2, 2) is divided by the points Q in the ratio 1 : 2. Does the line x – 2y = 0 contain Q?
Find the point of intersection of the lines 4x + 3y = 1 and 3x − y + 9 = 0. If this point lies on the line (2k – 1) x – 2y = 4; find the value of k.
Find the equation of the line passing through : (0, 1) and (1, 2)
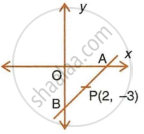
A and B are two points on the x-axis and y-axis respectively. P(2, −3) is the mid point of AB. Find the
- co-ordinates of A and B
- slope of line AB
- equation of line AB.
Find the value of a line parallel to the following line:
`"x"/4 +"y"/3` = 1
Find the equation of a line passing through (-5,-1) and perpendicular to the 3x + y = 9
Given equation of line L1 is y = 4.
(i) Write the slope of line, if L2 is the bisector of angle O.
(ii) Write the coordinates of point P.
(iii) Find the equation of L2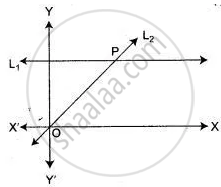
A and B are two points on the x-axis and y-axis respectively.

- Write down the coordinates of A and B.
- P is a point on AB such that AP : PB = 1 : 1.
Using section formula find the coordinates of point P. - Find the equation of a line passing through P and perpendicular to AB.
