Advertisements
Advertisements
प्रश्न
Find the values of a and b, if the sum of all the frequencies is 120 and the median of the following data is 55.
| Marks | 30 – 40 | 40 – 50 | 50 –60 | 60 – 70 | 70 –80 | 80 – 90 |
| Frequency | a | 40 | 27 | b | 15 | 24 |
उत्तर
Given: Median of observations = 55
And sum of frequencies = 120
| Marks `(f_i)` |
Frequency `(c.f.)` |
Cumulative frequency |
| 30 – 40 | a | a |
| 40 – 50 | 40 | a + 40 |
| 50 – 60 | 27 | a + 67 |
| 60 – 70 | b | a + b + 67 |
| 70 – 80 | 15 | a + b + 82 |
| 80 – 90 | 24 | a + b + 106 |
Now, `sumf_i` = 120 ......(Given)
⇒ a + b + 106 = 120
⇒ a + b = 14 ......(i)
Also, Median = 55
∴ Median class = 50 – 60
So, L = 50, h = 10, f = 27, c.f. = a + 40
Median = `L + ((N/2 - c.f.)/f) xx h`
55 = `50 + ((120/2 - (a + 40))/27) xx 10`
55 – 50 = `((60 - (a + 40))/27) xx 10`
5 = `((20 - a)/27) xx 10`
5 × 27 = 200 – 10a
135 = 200 – 10a
10a = 65
a = 6.5
Putting the value of a in equation (i), we get
6.5 + b = 14
b = 14 – 6.5 = 7.5
Hence, a = 6.5 and b = 7.5.
APPEARS IN
संबंधित प्रश्न
The lengths of 40 leaves of a plant are measured correct to the nearest millimeter, and the data obtained is represented in the following table:
| Length (in mm) | Number of leaves |
| 118 − 126 | 3 |
| 127 – 135 | 5 |
| 136 − 144 | 9 |
| 145 – 153 | 12 |
| 154 – 162 | 5 |
| 163 – 171 | 4 |
| 172 – 180 | 2 |
Find the median length of the leaves.
(Hint: The data needs to be converted to continuous classes for finding the median, since the formula assumes continuous classes. The classes then change to 117.5 − 126.5, 126.5 − 135.5… 171.5 − 180.5)
For a certain frequency distribution, the values of Assumed mean (A) = 1300, `sumf_id_i` = 900 and `sumfi` = 100. Find the value of mean (`barx`) .
The mean of following numbers is 68. Find the value of ‘x’. 45, 52, 60, x, 69, 70, 26, 81 and 94. Hence, estimate the median.
An incomplete distribution is given as follows:
| Variable: | 0 - 10 | 10 - 20 | 20 - 30 | 30 - 40 | 40 - 50 | 50 - 60 | 60 - 70 |
| Frequency: | 10 | 20 | ? | 40 | ? | 25 | 15 |
You are given that the median value is 35 and the sum of all the frequencies is 170. Using the median formula, fill up the missing frequencies.
The following table gives the frequency distribution of married women by age at marriage:
| Age (in years) | Frequency |
| 15-19 | 53 |
| 20-24 | 140 |
| 25-29 | 98 |
| 30-34 | 32 |
| 35-39 | 12 |
| 40-44 | 9 |
| 45-49 | 5 |
| 50-54 | 3 |
| 55-59 | 3 |
| 60 and above | 2 |
Calculate the median and interpret the results.
Compute the median for the following data:
| Marks | No. of students |
| More than 150 | 0 |
| More than 140 | 12 |
| More than 130 | 27 |
| More than 120 | 60 |
| More than 110 | 105 |
| More than 100 | 124 |
| More than 90 | 141 |
| More than 80 | 150 |
In a hospital, the ages of diabetic patients were recorded as follows. Find the median age.
| Age (in years) |
0 – 15 | 15 – 30 | 30 – 45 | 45 – 60 | 60 - 75 |
| No. of patients | 5 | 20 | 40 | 50 | 25 |
Compute mean from the following data:
| Marks | 0 – 7 | 7 – 14 | 14 – 21 | 21 – 28 | 28 – 35 | 35 – 42 | 42 – 49 |
| Number of Students | 3 | 4 | 7 | 11 | 0 | 16 | 9 |
The following table shows the daily wages of workers in a factory:
| Daily wages in (Rs) | 0 – 100 | 100 – 200 | 200 – 300 | 300 – 400 | 400 – 500 |
| Number of workers | 40 | 32 | 48 | 22 | 8 |
Find the median daily wage income of the workers.
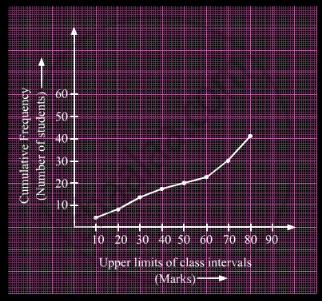
A student draws a cumulative frequency curve for the marks obtained by 40 students of a class as shown below. Find the median marks obtained by the students of the class.
If the median of the data: 6, 7, x − 2, x, 17, 20, written in ascending order, is 16. Then x=
The prices of different articles and demand for them is shown in the following frequency distribution table. Find the median of the prices.
|
Price (Rupees)
|
20 less than | 20 – 40 | 40 – 60 | 60 – 80 | 80 – 100 |
| No. of articles | 140 | 100 | 80 | 60 | 20 |
Find the median of the following frequency distribution:
| x | 10 | 11 | 12 | 13 | 14 | 15 |
| f | 1 | 4 | 7 | 5 | 9 | 3 |
Calculate the median of the following distribution:
| No. of goals | 0 | 1 | 2 | 3 | 4 | 5 |
| No. of matches | 2 | 4 | 7 | 6 | 8 | 3 |
Mode and mean of a data are 12k and 15A. Median of the data is ______.
The maximum bowling speeds, in km per hour, of 33 players at a cricket coaching centre are given as follows:
| Speed (km/h) | 85 – 100 | 100 – 115 | 115 – 130 | 130 – 145 |
| Number of players | 11 | 9 | 8 | 5 |
Calculate the median bowling speed.
The median of the following frequency distribution is 35. Find the value of x.
| Class: | 0 – 10 | 10 – 20 | 20 – 30 | 30 – 40 | 40 – 50 |
| Frequency: | 6 | 3 | x | 12 | 19 |
Using the empirical relationship between the three measures of central tendency, find the median of a distribution, whose mean is 169 and mode is 175.
Find the modal and median classes of the following distribution.
| Class | 0 – 20 | 20 – 40 | 40 – 60 | 60 – 80 | 80 – 100 |
| Frequency | 11 | 22 | 19 | 18 | 7 |
The median of first 10 natural numbers is ______.
