Advertisements
Advertisements
प्रश्न
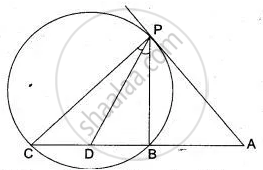
From the given figure, prove that : AP + BQ + CR = BP + CQ + AR.

Also show that : AP + BQ + CR = `1/2` × Perimeter of ΔABC.
उत्तर
Since from B, BQ and BP are the tangents to the circle
Therefore, BQ = BP ...(i)
Similarly, we can prove that
AP = AR ...(ii)
And CR = CQ ...(iii)
Adding,
AP + BQ + CR = BP + CQ + AR ...(iv)
Adding AP + BQ + CR to both sides
2(AP + BQ + CR) = AP + PQ + CQ + QB + AR + CR
2(AP + BQ + CR) = AB + BC + CA
Therefore, AP + BQ + CR = `1/2` × (AB + BC + CA)
AP + BQ + CR = `1/2` × perimeter of triangle ABC
APPEARS IN
संबंधित प्रश्न
The radius of a circle is 8 cm. calculate the length of a tangent draw to this circle from a point at a distance of 10 cm from its centre.
In the following figure; If AB = AC then prove that BQ = CQ.

Tangents AP and AQ are drawn to a circle, with centre O, from an exterior point A. Prove that : ∠PAQ = 2∠OPQ
PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate:
- ∠CBT
- ∠BAT
- ∠APT

In the following figure, PQ is the tangent to the circle at A, DB is the diameter and O is the centre of the circle. If ∠ADB = 30° and ∠CBD = 60°, calculate:
- ∠QAB,
- ∠PAD,
- ∠CDB.

AB is the diameter and AC is a chord of a circle with centre O such that angle BAC = 30°. The tangent to the circle at C intersects AB produced in D. show that BC = BD.
In the given figure, AC = AE. Show that:
- CP = EP
- BP = DP

In Fig. AP is a tangent to the circle at P, ABC is secant and PD is the bisector of ∠BPC. Prove that ∠BPD = `1/2` (∠ABP - ∠APB).
A, B, and C are three points on a circle. The tangent at C meets BN produced at T. Given that ∠ ATC = 36° and ∠ ACT = 48°, calculate the angle subtended by AB at the center of the circle.
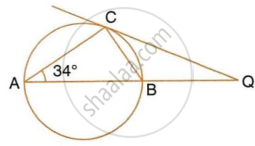
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q. If ∠CAB = 34°, find:
- ∠CBA
- ∠CQB