Advertisements
Advertisements
प्रश्न
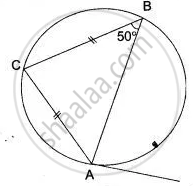
PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate:
- ∠CBT
- ∠BAT
- ∠APT

उत्तर

Join AT and BT.
i. TC is the diameter of the circle
∴ ∠CBT = 90° ...(Angle in a semi-circle)
ii. ∠CBA = 70°
∴ ∠ABT = ∠CBT – ∠CBA
= 90° – 70°
= 20°
Now, ∠ACT = ∠ABT = 20° ...(Angle in the same segment of the circle)
∴ ∠TCB = ∠ACB – ∠ACT
= 50° – 20°
= 30°
But, ∠TCB = ∠TAB ...(Angles in the same segment of the circle)
∴ ∠TAB or ∠BAT = 30°
iii. ∠BTX = ∠TCB = 30° ...(Angles in the same segment)
∴ ∠PTB = 180° – 30° = 150°
Now in ΔPTB
∠APT + ∠PTB + ∠ABT = 180°
`=>` ∠APT + 150° + 20° = 180°
`=>` ∠APT = 180° – (150° + 20°)
`=>` ∠APT = 180° – 170° = 10°
APPEARS IN
संबंधित प्रश्न
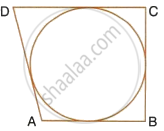
If the sides of a parallelogram touch a circle in following figure, prove that the parallelogram is a rhombus.
From the given figure, prove that : AP + BQ + CR = BP + CQ + AR.

Also show that : AP + BQ + CR = `1/2` × Perimeter of ΔABC.
From a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that:
∠AOP = ∠BOP
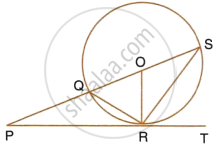
In the given figure, PT touches the circle with centre O at point R. Diameter SQ is produced to meet the tangent TR at P. Given ∠SPR = x° and ∠QRP = y°;
Prove that:
- ∠ORS = y°
- write an expression connecting x and y.
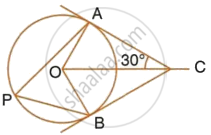
In the given figure, O is the centre of the circumcircle ABC. Tangents at A and C intersect at P. Given angle AOB = 140° and angle APC = 80°; find the angle BAC.

In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If ∠ACO = 30°, find:
- ∠BCO
- ∠AOB
- ∠APB
In the given figure, XY is the diameter of the circle and PQ is a tangent to the circle at Y.
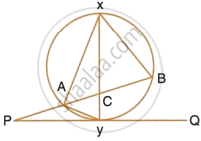
If ∠AXB = 50° and ∠ABX = 70°, find ∠BAY and ∠APY.
In the given figure, PT touches a circle with centre O at R. Diameter SQ when produced to meet the tangent PT at P. If ∠SPR = x° and ∠QRP = y°; Show that x° + 2y° = 90°

In Fig. AP is a tangent to the circle at P, ABC is secant and PD is the bisector of ∠BPC. Prove that ∠BPD = `1/2` (∠ABP - ∠APB).
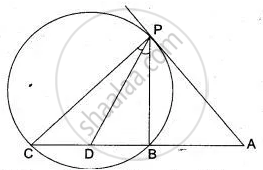
In Fig. AT is a tangent to the circle. If m∠ABC = 50°, AC = BC, Find ∠BAT.