Advertisements
Advertisements
प्रश्न
If a line is drawn parallel to the base of an isosceles triangle to intersect its equal sides, prove that the quadrilateral so formed is cyclic.
उत्तर
Given: ΔABC is an isosceles triangle such that AB = AC and also DE || BC.
To prove: Quadrilateral BCDE is a cyclic quadrilateral.
Construction: Draw a circle passes through the points B, C, D and E.
Proof: In ΔABC, AB = AC ...[Equal sides of an isosceles triangle]
⇒ ∠ACB = ∠ABC ...(i)
Since, DE || BC ...[Angles opposite to the equal sides are equal]
⇒ ∠ADE = ∠ACB [Corresponding angles] ...(ii)
On adding both sides by ∠EDC in equation (ii), we get
∠ADE + ∠EDC = ∠ACB + ∠EDC
⇒ 180° = ∠ACB + ∠EDC ...[∠ADE and ∠EDC from linear pair axiom]
⇒ ∠EDC + ∠ABC = 180° ...[From equation (i)]
Hence, BCDE is a cyclic quadrilateral, because sum of the opposite angles is 180°.
APPEARS IN
संबंधित प्रश्न
ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠CAD = ∠CBD.
AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
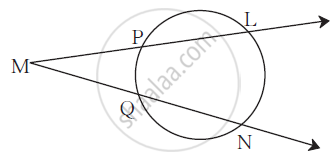
In the figure m(arc LN) = 110°,
m(arc PQ) = 50° then complete the following activity to find ∠LMN.
∠ LMN = `1/2` [m(arc LN) - _______]
∴ ∠ LMN = `1/2` [_________ - 50°]
∴ ∠ LMN = `1/2` × _________
∴ ∠ LMN = __________
In the given figure, ∠BAD = 78°, ∠DCF = x° and ∠DEF = y°. Find the values of x and y.

ABCD is a cyclic quadrilateral in BC || AD, ∠ADC = 110° and ∠BAC = 50°. Find ∠DAC.
ABCD is a cyclic quadrilateral in ∠DBC = 80° and ∠BAC = 40°. Find ∠BCD.
ABCD is a cyclic quadrilateral in ∠BCD = 100° and ∠ABD = 70° find ∠ADB.
In the given figure, ABCD is a cyclic quadrilateral in which AC and BD are its diagonals. If ∠DBC = 55° and ∠BAC = 45°, find ∠BCD.
ABCD is a cyclic quadrilateral such that ∠ADB = 30° and ∠DCA = 80°, then ∠DAB =
PQRS is a cyclic quadrilateral such that PR is a diameter of the circle. If ∠QPR = 67° and ∠SPR = 72°, then ∠QRS =
