Advertisements
Advertisements
प्रश्न
if `sec A = 5/4` verify that `(3 sin A - 4 sin^3 A)/(4 cos^3 A - 3 cos A) = (3 tan A - tan^3 A)/(1- 3 tan^2 A)`
उत्तर
We have
`sec A = 5/4`

In ΔABC
`AC^2 = AB^2 + BC^2`
`=> (5)^2 = (4)^2 + (BC)^2`
`=> BC^2 = 25 - 16`
=> BC = 3
`sin A = 3/5, cos A = 4/5 and tan A = 3/4`
Now
`(3sin A - 4sin^3 A)/(4 cos^3 A - 3 cosA) = (3 tan A - tan^3 A)/(1 - 3tan^2 A)`
`=> (3 xx 3/5 - 4 xx (3/5)^2)/(4 xx (4/5)^3 - 3 xx (4/5)) = (3xx (3/4) - (3/4)^3)/(1 - 3xx(3/4)^2)`
`=> (9/5 - 108/125)/(256/25 - 12/5) = (9/4 - 27/64)/(1 - 27/16)`
`=> ((225 - 108)/125)/((256 - 300)/125) = ((144 - 27)/64)/((16 - 27)/16)`
`=> 117/(-44) = 117/(-11xx4)`
=> L.H.S = R.H.S
APPEARS IN
संबंधित प्रश्न
If θ = 30° verify `tan 2 theta = (2 tan theta)/(1 - tan^2 theta)`
If Sin (A + B) = 1 and cos (A – B) = 1, 0° < A + B ≤ 90° A ≥ B. Find A & B
In ∆PQR, right-angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠P and ∠R.
If 3tan θ 4 , show that `((4cos theta - sin theta ))/((4 cos theta + sin theta))=4/5`
Evaluate:
`(sin^2 30^0 + 4 cot^2 45^0-sec^2 60^0)(cosec^2 45^0 sec^2 30^0)`
If sin (A + B) = 1 and cos (A – B) = 1, 00 ≤ (A + B) ≤ 900 and A > B, then find A and B.
If sin A = cos A, find the value of 2 tan2A - 2 sec2 A + 5.
In an isosceles triangle ABC, AB = BC = 6 cm and ∠B = 90°. Find the values of cos2 C + cosec2 C
In a right-angled triangle PQR, ∠PQR = 90°, QS ⊥ PR and tan R =`(5)/(12)`, find the value of tan ∠SQR
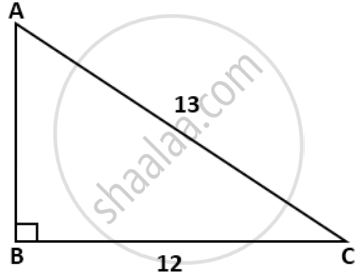
In the given figure, AC = 13cm, BC = 12 cm and ∠B = 90°. Without using tables, find the values of: sin A cos A