Advertisements
Advertisements
प्रश्न
if `sin theta = 3/4` prove that `sqrt(cosec^2 theta - cot)/(sec^2 theta - 1) = sqrt7/3`
उत्तर
We have `sin theta = 3/4`
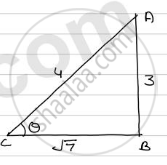
In ΔABC
`AC^2 = AB^2 + BC^2`
`=> (4)^2 = (3)^2 + BC^2`
`=> BC^2= 16 - 9`
`=> BC^2 = 7`
`=> BC = sqrt7`
`:. cosec theta = 4/3, sec theta = 4/sqrt7 and cot theta = sqrt7/3`
Now
L.H.S `sqrt((cosec^2 theta - cot^2 theta)/(sec^2 theta - 1))`
`= sqrt(((4/3)^2 - (sqrt7/3)^2)/((4/sqrt7)^2 - 1)`
`= sqrt((16/9 - 7/9)/(16/7 - 1)`
`=sqrt((9/9)/((16 - 7)/7 ))`
`= sqrt(7/9)`
`= sqrt7/3`
= R.H.S
APPEARS IN
संबंधित प्रश्न
State whether the following are true or false. Justify your answer.
cot A is the product of cot and A.
State whether the following are true or false. Justify your answer.
sin θ = `4/3`, for some angle θ.
Prove that `(sin "A" - 2sin^3 "A")/(2cos^3 "A" - cos "A") = tan "A"`
In the following, one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios.
`cos theta = 12/2`
Evaluate the following
sin 45° sin 30° + cos 45° cos 30°
Evaluate the following
cos 60° cos 45° - sin 60° ∙ sin 45°
Find the value of x in the following :
`2 sin x/2 = 1`
If sin (A − B) = sin A cos B − cos A sin B and cos (A − B) = cos A cos B + sin A sin B, find the values of sin 15° and cos 15°.
Prove that: cot θ + tan θ = cosec θ·sec θ
Proof: L.H.S. = cot θ + tan θ
= `square/square + square/square` ......`[∵ cot θ = square/square, tan θ = square/square]`
= `(square + square)/(square xx square)` .....`[∵ square + square = 1]`
= `1/(square xx square)`
= `1/square xx 1/square`
= cosec θ·sec θ ......`[∵ "cosec" θ = 1/square, sec θ = 1/square]`
= R.H.S.
∴ L.H.S. = R.H.S.
∴ cot θ + tan θ = cosec·sec θ
If f(x) = `3cos(x + (5π)/6) - 5sinx + 2`, then maximum value of f(x) is ______.
